Consider the function
$$f_{n}(x)=e^{-x^2}x^n.$$
and the function
$$h_p(x):=e^{-\vert x \vert^p}.$$
My goal is to analyze
$$ F_p(y):=\frac{(f_2*h_p)(y)}{(f_0*h_p)(y)}- \left(\frac{(f_1*h_p)(y) }{(f_0*h_p)(y)}\right)^2$$
Question: Can we show that $F_p$ has a global maximum at zero for $p>2$ and a global minimum at zero for $p<2$?
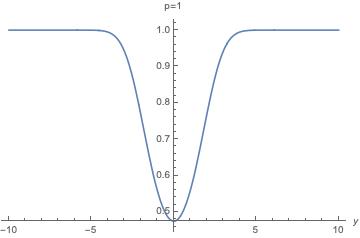
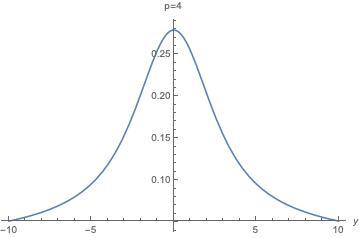
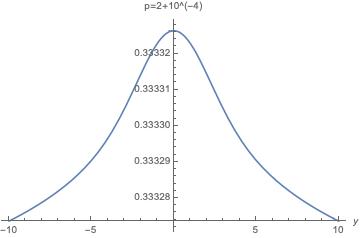
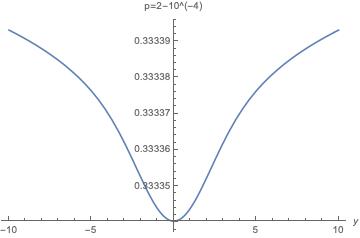
Here are graphs from Mathematica for
- $F_1$, with a unique minimum
- $F_4$, with a unique maximum
- $F_{2+10^{-4}}$, i.e. with an exponent slightly above $2$
- $F_{2-10^{-4}}$, i.e. with an exponent slightly below $2$
Further observations:
Iosif Pinelis showed that $F_2(y)$ is constant, see this earlier question of mine [question I asked some days ago][1]question I asked some days ago.
All functions $F_p$ are positive by the Cauchy-Schwarz inequality.
The functions $F_p$ are not log-convex or log-concave in general.
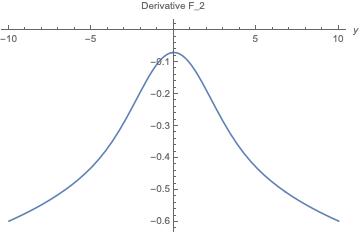
Finally, I computed the first derivative for convolving with $e^{-\vert x \vert^p}$ at $p=2$ by numerically differentiating
If you have any other conjectures you would like to me to verify, I am happy to do so. Just leave a comment! [1]: https://i.sstatic.net/2NvRL.jpg [2]: https://i.sstatic.net/VIFOi.jpg [3]: https://i.sstatic.net/H1N0U.jpg [4]: https://i.sstatic.net/4HMAP.jpg [5]: https://i.sstatic.net/Caurg.jpg [6]: https://i.sstatic.net/0uVdR.jpg