It is well-known that any two great circles intersects on a sphere. In fact, there are infinitely many half great circles can be drawn on a sphere with a common intersection. Intuitively, it seems to me that there is only finitely many half great circles can be drawn on a sphere if one do not allow intersection.
I try to investigate this by considering stereographic projection.
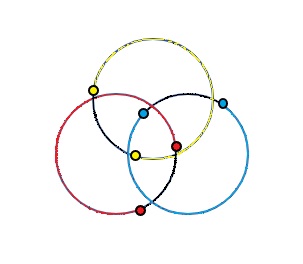
I came up with the above picture in which the yellow edge, blue edge and red edge on a plane correspond to the three geodesics of length $\ge \pi$ on the sphere. Since there is no intersection among the yellow edge, blue edge and red edge, the three geodesics do not intersect either.
In other words, this picture shows that $3$ geodesics of length $\ge \pi$ can be drawn on a sphere without any intersection. In particular, this implies that $3$ half great circles can be drawn on a sphere without any intersection.
If one draw the fourth circle on top of the three, it seems impossible to draw a fourth edge on the circle which corresponds to a geodesic of length $\ge \pi$ on the sphere.
Conjecture:
The maximum number of half great circles can be drawn on a sphere is $3$ if one do not allow intersection.
How to prove/disprove this conjecture rigorously?
