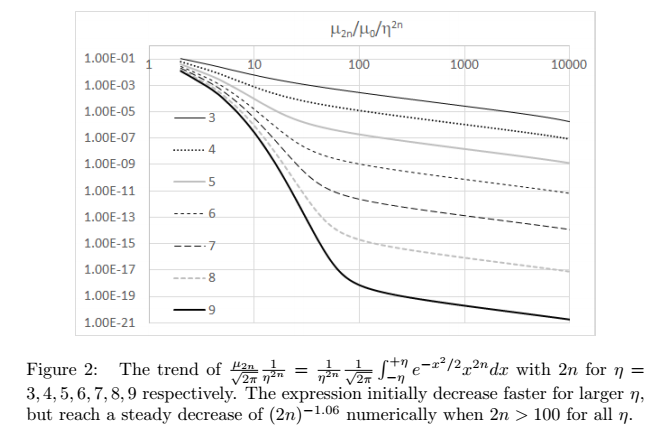
Is there an analytic solution or approximation for the following Gaussian-like integration? $\frac{1}{\eta^{2n}} \frac{1}{\sqrt{2 \pi}} \int_{-\eta}^{+\eta} e^{-x^2/2} x^{2n} dx$? The numerical plot suggests that it initially decrease faster, but reach a steady decrease of $(2n)^{-1.06}$ numerically when $2n > 100$ for all $\eta$.
Became Hot Network Question