Let $k$ be positive integer, not a square and let $u,v$$u_k,v_k$ be non-trivial solutions to the Pell equation $u^2-k^3 v^2=\pm 1$$u_k^2-k^3 v_k^2=\pm 1$.
Q1 How small $u$$u_k$ can be infinitely often in terms ofas function $k$?
This gives rise to the good abc triple $(u^2,-k^3 v^2,\mp 1)$$(u_k^2,-k^3 v_k^2,\mp 1)$ so abc implies $u$$u_k$ can't be too small, it can't be $\log(u)=C\log(k)$$\log(u_k)=C\log(k)$.
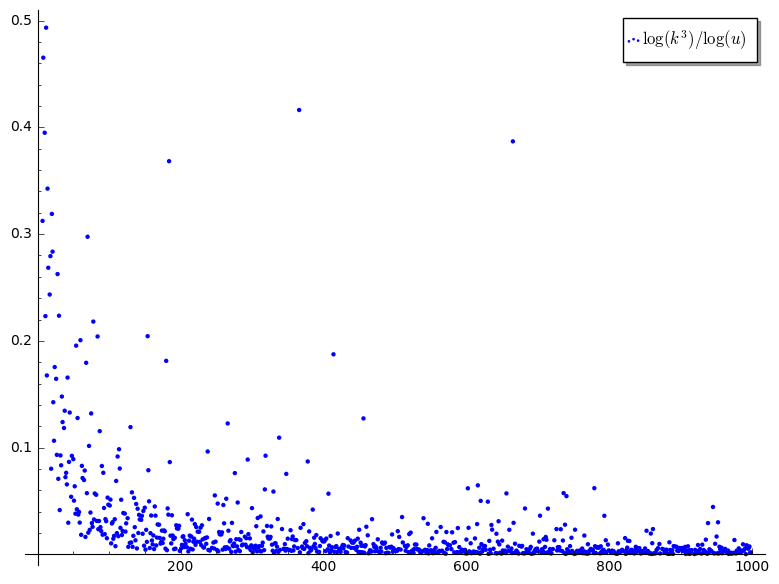
$k/\log(u)$$k/\log(u_k)$ appears to diverge.
$\log^2(k^3) / \log(u)$$\log^2(k^3) / \log(u_k)$ appears better, with large sporadic peaks. $\log(k^3)/\log(u)$$\log(k^3)/\log(u_k)$ doesn't appear to go to zero fast and also have sporadic peaks.
Q2 for positive constants $C_0, 1 \le C < 2$ do we have $u_k<C_0 \exp(\log^C(k^3))$
Possible approach is to find $\sqrt{k^3}$ with relatively short continued fraction expansion.
We believe the set of small $u$$u_k$ to be very sparse, so the numerical evidence might be misleading.
$k$ is on the $x$ axis: