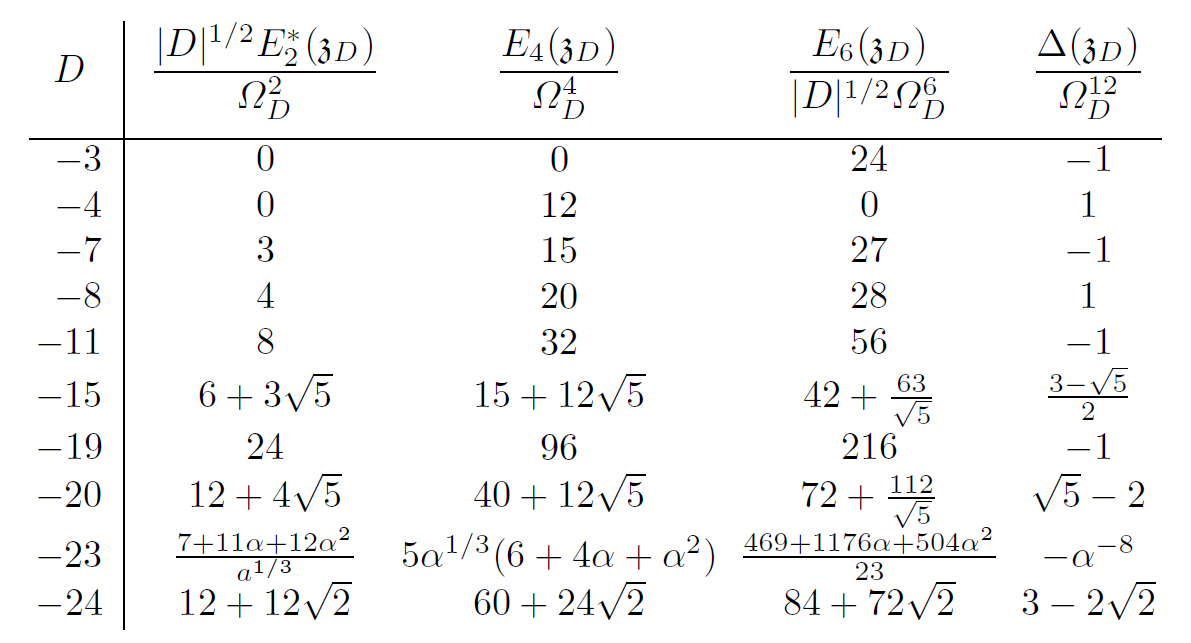
I'm studying these algebraic numbers in the table below and have succesfully shown what i wanted with the given values I had.
The table is found in the book "The 1-2-3 of Modular Forms" by Jan Hendrik Bruinier, Gerard van der Geer, Günter Harder and Don Zagier.
I am looking for a way to calculate more numbers or/and just more values for other discriminants (-31,-35,-39,-40,-43,...).
I hope someone can help.
![Table of algebraic numbers][1]
[1]: https://i.sstatic.net/r9JZc.png