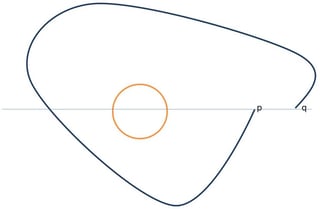
But the same is true if we keep the closed geodesic $\gamma_1$, the innermost one, and replace the smooth closed geodesic $\gamma_2$, the outermost one, by a non smooth one denoted again by $\gamma_2$ provided $\angle p+\angle q =2\pi$. (Please see the figure bellow). In fact $\gamma_2$ is the union of two pieces of geodesics whose union make a simple closed curve which can be counted as a 2-polygon(with two vertex $p,q$), whose sum angles is $2\pi$, look at the following figure(Note that the horizontal axis is assumed to be a geodesic as well as other curves in the figurefigure> Furthermore it is obvious that by "angle" we mean Riemannian angle, that is the angles $\angle p$ and $\angle q$ are Riemannian angles not the Euclidean angle drown in the picture ):