Consider the Stirling numbers of the first kind $s(i, j)$ , and form a matrix $S_1(n),$ where the $i, j$$(i, j)$th entry is $s(i, j)$. (IMPORTANT NOTE the indices start at $0,$ so this matrix is $(n+1)\times (n+1).$) This matrix is the inverse of the similarcorresponding matrix for the Stirling numbers of the second kind, and the question applies to both of them.
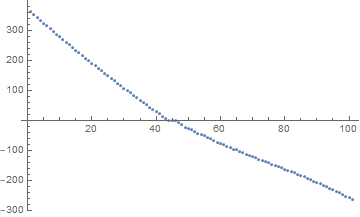
Now, for the question: I have computed the logs of the singular values of these things (you have to do this to high precision, since the entries grow very fast). Here is the plot of the singular values for $n=100:$
You will note two empirical facts: firstly, $1$ is a singular value, and secondly the singular values bigger than one$1$ decay exponentially, as do the ones smaller than one$1$, but with a different rate. Has this been observed? Can one prove it (or at least explain it)?
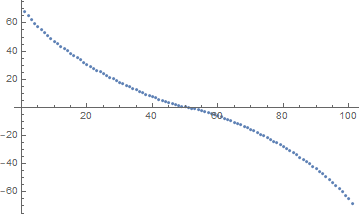
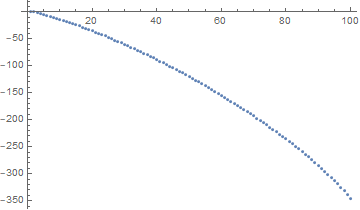
ADDITION In my comment on the answer, I speak of the shape of the curve, to. To underscore what I mean, here are two more interesting matrices: the binomial coefficient matrix binomial coefficient matrix (which should be, in principle, similar to the Stirling matrix, due to the recurrence relation), and the Hilbert matrixHilbert matrix. Here are the singular value plots of both (in order), again logarithmically. You will note that all three curves are regular,. While the Stirling curve is piecewise linear, the Binomial curve is convex (on the top segment) and the Hilbert curve is concave. This must mean something :)