_________
 |         |
 |     ___ |
 |____|  |_|

This is just a made up-shape whose cardinality could be computed by such gluing axioms, if we can get the two sets of axioms to agree.

What is counter-intuitive, is that both theories focus on the edges rather than the squares and don't really care about dominoes, but instead about counting perfect matchings.

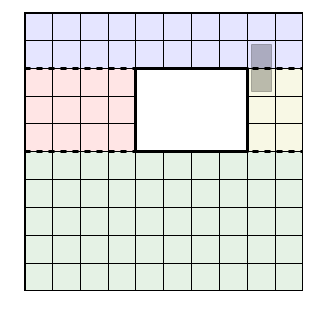
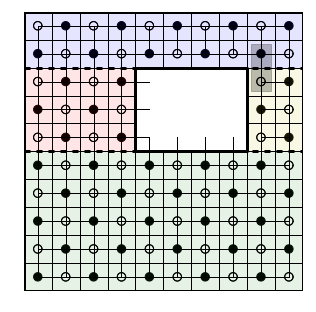
Here is a shape of a deficient rectangle. And I split into 4 rectangles and for each region I can count the tilings. We are not done because it's possible to have dominoes cross the border slightly.

CR Cimasoni-Reshetikhin says (among many other things), that you define a matrix indexed by the edges that cross the boundary, and you "resolve" each one. Either there's domino there or not... So in this way, if you "glue" the two rectangles together, you have to glue $2^n$ nearby shapes, where $n$ counts the number of border edges.

They obtain some formula which is phrased in terms of the Arf invariant of the Spin structure.

$$ Z(\Gamma) = \frac{1}{2^g} \sum_{[\xi] \in \mathcal{S}(\Sigma) } \text{Arf}(\xi) \; \text{Pf} (A^\Gamma)$$ where $[\xi]$ is a spin structure on the surface $\Sigma$ and $A^\Gamma$ is the Kasteleyn matrix. In a way, the Kasteleyn orientation is like a discrete spin structure.

GK Gaiotto-Kapustin define a topological invariant, so possibly we have ruled out the dimer model. Nor does [CR] claim to have a topological invariant. In any case, we can triangulate a manifold $X$ (in our case, the rectangle $$ X = \Big( [0,10]\times [0,10] \Big) \backslash \Big( [4,8] \times [5,8] \Big) $$ So we should define a triangulation on the dual graph.

The definition in [GK] should agree with what I've discussed before, I've practically outlined what it should be. It is a weighted sum over various "acceptable" . If we choose $A$ correctly and the weights $C_{ijk}$ and $g_{ij} = C_{ik}^l C_{jl}^k$, then perhaps we get a count of the domino tilings.

The answer to my question is "yes". Technically, it's a spin-TQFT but now I am trying to make sense of that answer.

• Dimers on surface graphs and spin structures. I David Cimasoni, Nicolai Reshetikhin
• Dimers on surface graphs and spin structures. II David Cimasoni, Nicolai Reshetikhin

"Quantum Field Theory" means a lot of things to a lot of different people. Here is their definition (a modification of the Atiyah-Segal axioms)

A quantum field theory on graphs

• fix a finite dimensional vector space $V$
• To the oriented boundary $\partial \Gamma$ assign the vector space $$ Z(\partial \Gamma) = \bigotimes V \otimes \bigotimes V^* $$ where $V$ is the vector space dual to $V$.
• To a finite graph $\Gamma$ with with oriented bounardy $\partial \Gamma$ and weight system $w$ assign some vector $Z(\Gamma; w) \in Z(\partial \Gamma) $ with $Z(\varnothing; w) = 1 \in \mathbb{C} = Z(\varnothing)$

A broader less careful definition could be find in the discussion of Gaiotto and Kapustin from 2015. [GK]:

An oriented 2d TQFT can be defined axiomatically as a functor from a geometric category $\text{Cob}_2$ to the category of vector spaces ($\mathbf{Vect}$). The category $\text{Cob}_2$ has closed 1-manifolds as objects and oriented bordisms between them as morphisms. It is well-known there is a 1-1 correspondence between 2d TQFTs and commutative Frobenius algebras...

If you step back, these definitions look compatible. Perhaps I have to be careful about the notion of "cobordism" but there is a lot of discussion about that. I am more concerned with getting either Cimasoni-Reshethikhin or Gaiotto-Kapustin definitions to produce a number and getting them to (nearly) agree.

There are really only few dimer examples that are computed too carefully. We know the number of tilings of a rectangle is:

$$ \# (2m \times 2n) = \prod_{j=1}^m \prod_{k=1}^n \big( 4 \cos^2 \frac{\pi j}{2m+1} + 4 \cos^2 \frac{\pi k}{2n+1}\big)$$

This can be found by multiplying the eigenvalues of the Kasteleyn operator for the graph (a discrete Laplacian). And for the rectangle that's an easy shape.

I am not even sure the partition function is the same as the number of tilings. Whichever defiition you pick the TQFT structure is suggesting relations between the numbers of tilings of various shapes with boundary, the easiest of which to discuss would be rectangular shapes.

  _________
 |         |
 |     ___ |
 |____|  |_|

This is just a made up-shape whose cardinality could be computed by such gluing axioms, if we can get the two sets of axioms to agree.

The answer to my question is "yes". Technically, it's a spin-TQFT but now I am trying to make sense of that answer.

• Dimers on surface graphs and spin structures. I David Cimasoni, Nicolai Reshetikhin
• Dimers on surface graphs and spin structures. II David Cimasoni, Nicolai Reshetikhin

"Quantum Field Theory" means a lot of things to a lot of different people. Here is their definition (a modification of the Atiyah-Segal axioms)

A quantum field theory on graphs

• fix a finite dimensional vector space $V$
• To the oriented boundary $\partial \Gamma$ assign the vector space $$ Z(\partial \Gamma) = \bigotimes V \otimes \bigotimes V^* $$ where $V$ is the vector space dual to $V$.
• To a finite graph $\Gamma$ with with oriented bounardy $\partial \Gamma$ and weight system $w$ assign some vector $Z(\Gamma; w) \in Z(\partial \Gamma) $ with $Z(\varnothing; w) = 1 \in \mathbb{C} = Z(\varnothing)$

A broader less careful definition could be find in the discussion of Gaiotto and Kapustin from 2015. [GK]:

An oriented 2d TQFT can be defined axiomatically as a functor from a geometric category $\text{Cob}_2$ to the category of vector spaces ($\mathbf{Vect}$). The category $\text{Cob}_2$ has closed 1-manifolds as objects and oriented bordisms between them as morphisms. It is well-known there is a 1-1 correspondence between 2d TQFTs and commutative Frobenius algebras...

If you step back, these definitions look compatible. Perhaps I have to be careful about the notion of "cobordism" but there is a lot of discussion about that. I am more concerned with getting either Cimasoni-Reshethikhin or Gaiotto-Kapustin definitions to produce a number and getting them to (nearly) agree.

There are really only few dimer examples that are computed too carefully. We know the number of tilings of a rectangle is:

$$ \# (2m \times 2n) = \prod_{j=1}^m \prod_{k=1}^n \big( 4 \cos^2 \frac{\pi j}{2m+1} + 4 \cos^2 \frac{\pi k}{2n+1}\big)$$

This can be found by multiplying the eigenvalues of the Kasteleyn operator for the graph (a discrete Laplacian). And for the rectangle that's an easy shape.

I am not even sure the partition function is the same as the number of tilings. Whichever defiition you pick the TQFT structure is suggesting relations between the numbers of tilings of various shapes with boundary, the easiest of which to discuss would be rectangular shapes.

  _________
 |         |
 |     ___ |
 |____|  |_|

This is just a made up-shape whose cardinality could be computed by such gluing axioms, if we can get the two sets of axioms to agree.

Q Despite the answer being "yes" there are two possibly incompatible yes answers. One QFT definition depends on the choice of graph embedding, the other is an invariant on surfaces with boundary and does not depend on choice of triangulation. Traditionally the dimer model counts the number of perfect matchings. Even if we pick a rectangle, that region is topologically a disc.. So the TQFT invariant should not depend on our choice of rectangle.

Have I shown that Gaiotto-Kapustin and Cimasoni-Reshetikhin cannot agree?