Max Noether writes as if he knew more about Gordan's thoughts on infinitesimals than what he tells readers in the obituraryobituary.
Noether insinuates that Kronecker was present at the disputation.
I don't know what the regulations for disputations were in the 1860s at University of Berlin. (This is the 'ancestor' of what is by now one of at least three universities in Berlin.) If notes were taken, then they may have survived, though I think this unlikely. (And even if they have survived: anyone who knows what minutes of exams usually look like will not expect there to be much information in them. The best one could hope for is that Gordan later himself wrote carefully about infinitesimals.)
One could even hope that this question has (some sort of) mathematical answer; 155 years are not much by historians' standards. Of course, one should not expect there to be much in exam minutes, even conditioning on the, by itself, unlikely event that such were taken and have survived.
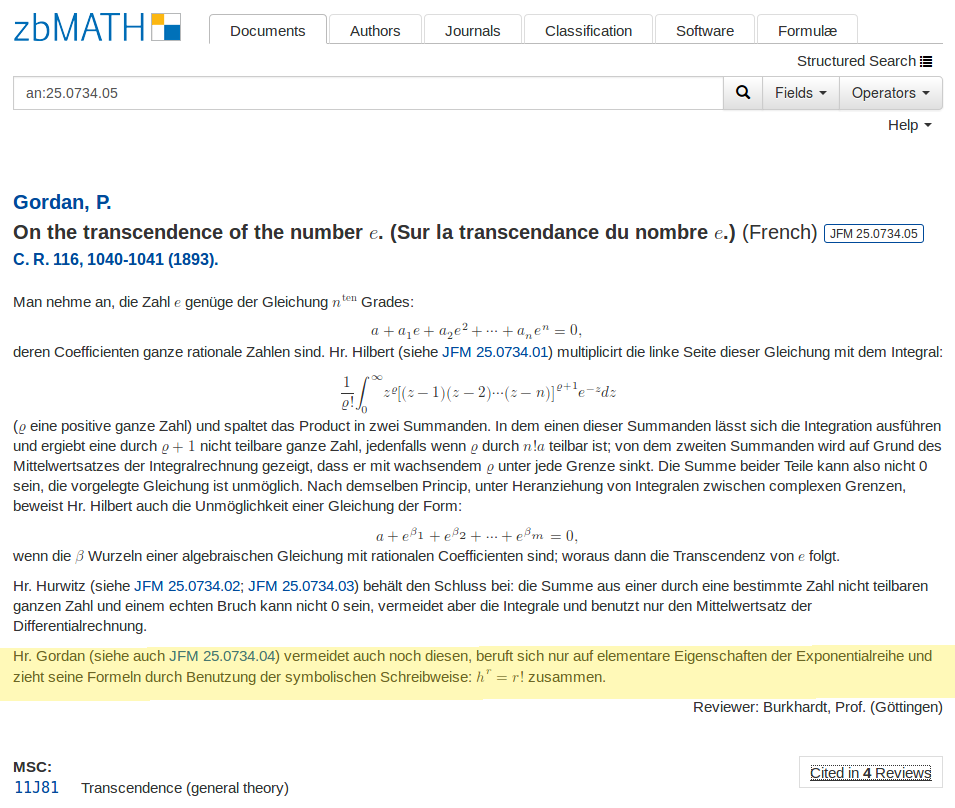
Only once did Gordan ever work on a non-algebraic topic: in 1893 Hilbert had, based on a new idea, given a simple proof of the transcendence of the numbers $e$ and $\pi$; and immediately afterwards Hurwitz had given a modification of the proof, by way of avoiding the integrals used by Hilbert, essentially by using integration-by-parts, and by the use of the simplest case of the intermediate-value-theorem. In an article (54), which by the way only had the aim of giving an exposition of Hurwitz's deduction, 'played backwards', Gordan took one more step in towards an elementary proof, by replacing also the still remaining differential quotients by use of the Taylor series of $e^x$. [emphasis added; by the way: what is nowadays the 'most' elementary proof that $\pi$ and $e$ are transcendental; the only proof I ever studied in detail used integrals, and, as such, limits] Regarding the $r!=h^r$ symbolism, which has been emphasized by various others after Gordan's paper, it must however be said that it does not play any further role in the proof than being a mere abbreviating notation, and that it moreover has simply been lifted from Hurwitz's note. The estimation of the vanishing remainder term, an estimate which is essential for the proof and was left out of the note (54), has been carried out by Gordan in 1900 in an unpublished notebook in Gordan's Nachlass. 3
- The 'MathSci-Net' and 'Zentralblatt' of the 19th century was the 'Jahrbuch über die Fortschritte der Mathematik'; therein, Heinrich Burkhardt reviewed Gordan's 'trancendent'transcendent note'; for convenience, since it is not entirely off-topic (it is 'Gordan on the non-algebraic'), I reproduce the review here (it is also something of a self-contained summary of Hilbert's proof, and as such, maybe of interest in and of itself):
The blue link in the yellow lead to a review which is (displayed to me as) empty.
1To my way of thinking, this is all rather expected and unsurprising and in line with the usual reputation of Gordan: all the subjects Noether says Gordan avoided are second-order theories; in particular, in the mechanics of the time variational principles had an important role, and variational principles involve quantifier over sets of functions, i.e., second-order quantifiers; 19th century mechanics/variational principles/principles of least action, etc. to me seem recognizably non-algebraic. Also, one could now very much overinterpret and say something about whether and how much infinitesimals help in complex analysis, and that there, perhaps, limits are more necessary than in real analysis, and Gordan avoided complex analysis because of this. This, however, is evidently an overinterpretation.
2 And to me, it seems evident that if there is any hope of a mathematical answer, then this hope comes from the possibility that Gordan might later have written about infinitesimals/used them for something in published printed work. However, unfortunately, I think this is unlikely.
3 It might be an interesting separate historical problem to locate this "unpublished" notebook. Please note, though, that I don't think this relevant for the question: what Noether describes sounds like a rather ordinary estimate of a remainder term, presumably by inequalities; it does not sound like a use that Gordan made of infinitesimals, which don't yield effective estimates anyway, as far as I know. One should be clear that there is no sign that Gordan ever recognizably used infinitesimals, except for his thesis defense.