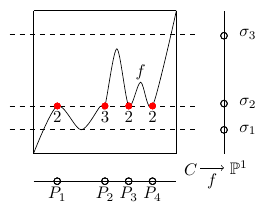
For a curve $C$ over a finite field, I am looking at the map $\phi: \operatorname{Hom}^d(C,\mathbb{P}^1) \to \operatorname{Sym}^d(C)$ where $\operatorname{Hom}^d(C,\mathbb{P}^1)$ are the functions of degree $d$ from $C \to \mathbb{P}^1$ in the $\operatorname{Hom}$-scheme $\operatorname{Hom}(C, \mathbb{P}^1)$, defined by $$\phi: f \mapsto [\sigma]\cdot \Gamma_f$$
i.e. we intersect the graph of $f$ in $C \times \mathbb{P}^1$ with the line $[\sigma]$ in $C \times \mathbb{P}^1$ (which is the inverse image of $\sigma$ under the projection to $\mathbb{P}^1$.
For example the $f$ in the picture would map to $\phi(f) = 2\cdot P_1 + 3\cdot P_2 + 2\cdot P_3 + 2\cdot P_4$
I have looked at Kollár's Rational Curves on Algebraic Varieties and Chapter 9 of Nakajima's Lectures on Hilbert Schemes of Points on Surfaces however I can't find the information I need of this map.
For example, is $\phi$ flat? What is the dimension of the fiber of $\phi$? Etc. Etc.
Does anyone know of a reference for this topic?
EDIT: It might be easier to dissectIf we take $\phi$: first$d > 2g$ we look at the graphcan view any point in $\Gamma_f$$\operatorname{Sym}^d(C)$ as an effective divisor $D$ of degree $f$. This we then intersect inside$d$ of $C \times \mathbb{P}^1$ with$C$. Then $[\sigma]$,$D$ is base-point free and so we getcan construct a map $d$ points$f: C \to \mathbb P^1$ with second coordinatefiber $\sigma$. Then we project onto$D$ above $\operatorname{Sym}^d(C)$ by losing that second coordinate$0$ (call this projectionor say, $\pi$$\sigma$), i.e So $\phi$ is surjective for $d > 2g$.
$$\operatorname{Hom}^d(C,\mathbb{P}^1) \to \operatorname{Hilb}(C\times \mathbb{P}^1) \to \operatorname{Hilb}^d(C\times \mathbb{P}^1) \to \operatorname{Sym}^d(C)$$ $$\qquad f \quad \mapsto \quad \Gamma_f \quad \mapsto \quad [\sigma]\cdot \Gamma_f\quad \mapsto \quad \pi([\sigma]\cdot \Gamma_f) $$ In general, I only care for $d$ much greater than 0 as I want to study it's behavior as $d$ grows.
