Looking at the images below, you recognize that the adjacency matrix of the graph $A_G$ splits up into three different colored submatrices, with $A_G=A_d+A_b+A_d$$A_G=A_r+A_b+A_d$ (where $d$ is dark, damn...).
It's obvious that $A_k^2=1$. Now have a look a the right coloring: You'll see that $(A_dA_r)^2=(A_rA_b)^2=(A_bA_d)^2=1$ as well. Let's use $a,b,c$ instead. We summarize this as:
$$
\langle a,b,c|a^2=b^2=c^2=(ab)^2=(bc)^2=(ca)^2=1\rangle =\Delta(2,2,2)
$$
This is a presentation of a triangle group $\Delta(2,2,2)$, a special kind of Coxeter group. The left image resembles the situation:
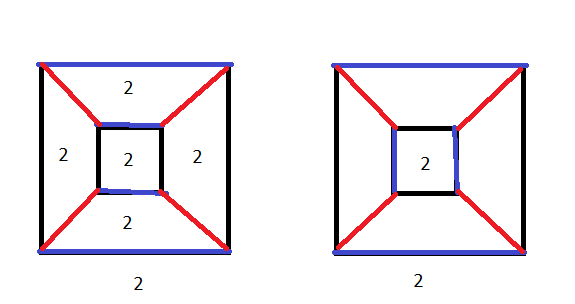
Now the right images deviates from the left at the inner square, where blue and dark are flipped; still a valid 3-edge coloring. It has Hamiltonian cycles (follow dark-red or red-blue edges), therefore its presentation is: $$ \langle a,b,c|a^2=b^2=c^2=(ab)^4=(bc)^4=(ca)^2=1 \rangle =\Delta(4,4,2) $$
So by flipping the inner cycle, we map $\Delta(2,2,2)\mapsto \Delta(4,4,2)$.
EDIT: This means that we change the classification from $\frac12+\frac12+\frac12=\frac32>1$, i.e. spherical, to $\frac14+\frac14+\frac12=1$, i.e. Euclidean.
Which branch of math deals with this mapping? What are good introductions to it?
Let's restrict to planar, bicubic graphs...
