I am following Ararat Babakhanian's Cohomological Methods in Group theory.
Let $A,B,C$ be $G$ modules then we have a $G$ module structre on $\text{Hom}_{\mathbb{Z}}(B,C)$ with $$\sigma.f(x)=\sigma(f\sigma^{-1}x)$$
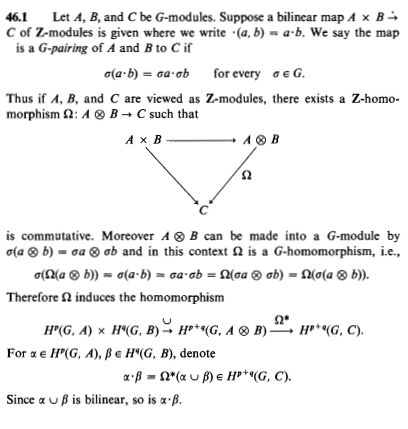
$ A\times B\xrightarrow{\cdot } C$ is called a $G$ pairing if $\sigma a\cdot \sigma b=\sigma (a\cdot b)$ for all $\sigma\in G$ and $a\in A, b\in B$.
By Universal property of tensor products, this induces a unique linear map $\Omega : A\otimes B\rightarrow C$ such that $\Omega (a\otimes b)=a\cdot b$.
There is then a homomorphism of cohomology groups $H^{p+q}(G,A\otimes B)\xrightarrow{\Omega^*} H^{p+q}(G,C)$ induced from $\Omega : A\otimes B\rightarrow C$.
We have cup product map $H^p(G,A)\times H^q(G,B)\xrightarrow{\cup} H^{p+q}(G,A\otimes B)$, composing with $\Omega^*$ we have the map $$H^p(G,A)\times H^q(G,B)\rightarrow H^{p+q}(G,C)$$
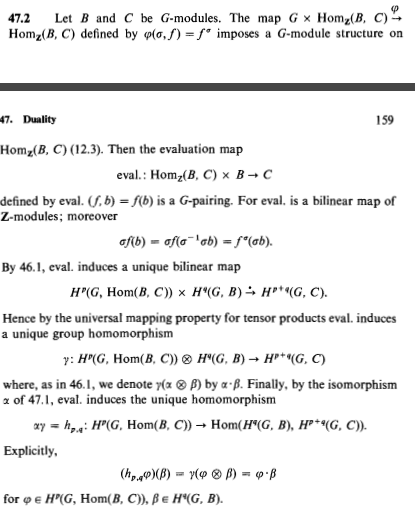
He then says we have evaluation (Bilinear) map $$\text{Hom}_{\mathbb{Z}}(B,C)\times B\rightarrow C$$
with $(f,b)\mapsto f(b)$. I checked that this is a $G$ pairing.
In this case we should get map
$$H^p(G,\text{Hom}_{\mathbb{Z}}(B,C) )\times H^q(G,B)\rightarrow H^{p+q}(G,C)$$
But then, he writes there is a map
$$H^p(G,\text{Hom}(B,C) )\times H^q(G,B)\rightarrow H^{p+q}(G,C)$$
Where $\text{Hom}(B,C)$ denote all $G$ module homomorphisms..
So, what happened here? Though We can restrict evaluation mp to $\text{Hom}(B,C)$, there is no module strutre on this, so we can not talk about cohomology or anything..
This continued in further material.. Let me know what is the point here.