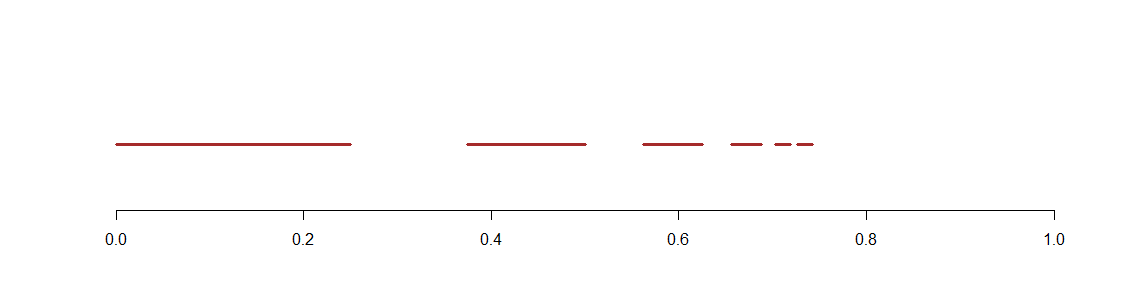
Say that we have a line $\left(0,0.5\right)$. I want a process that can split that line and half and move that half a bit, and then take half of that half and moved it and so on, so that by the end all the pieces of the line would still be within the region $\left(0,1\right)$.
An example of such a series is to take half of the line and move it half its size: $(0, 0.5)$
$(0, 0.25)$, $(0.375, 0.625)$
$(0, 0.25)$, $(0.375, 0.5)$, $(0.5625, 0.6875)$
- Is there a name for such a series? (it is similar to a cantor set, but not quite)
- How does one formally prove that this set will never reach 1, no matter how many splits we'll make?
Figure for 5 splits: