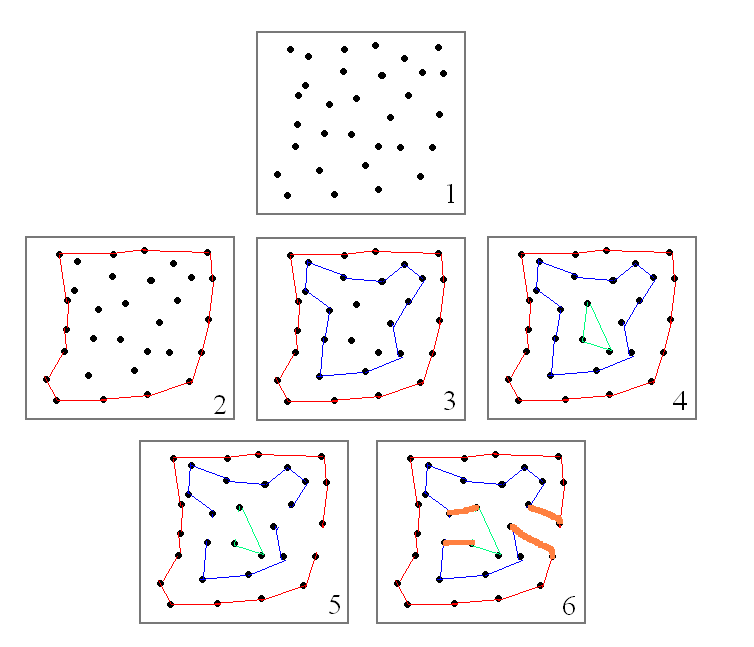
The answer is yes. firstFirst we draw a cycle with the outestoutmost vertices such that other vertices are within the cycle. (This is step 2 in above picture .) thenThen we repeat this for vertices that are not on the first cycle and draw the second cycle. weWe repeat this until all vertices lie on a unique simple closed cycle. Now we delete an edge from each two adjacent cycles and connecting vertices such that two cycles exchange in one longer cycle. thenThen repeat this until we have had a cycle consists all vertices.
