Earlier today, somebody asked what looks like a homework problem, but admits the following reading which I think is interesting:
Suppose $a_1,\dots, a_n$ are positive integers, and $\varepsilon$ is a positive real number which you can take to be as small as you like. How many non-overlapping unit hypercubes can you fit into an $n$-dimensional rectangular solid with side lengths $a_i-\varepsilon$?
It's clear that you can't fit $\prod_i a_i$ and that you can fit at least $\prod_i (a_i-1)$. A little playing around shows that you can sometimes fit strictly more than $\prod_i (a_i-1)$. For example, here's a packing of three unit squares into a $(2-\varepsilon)\times(3-\varepsilon)$ rectangle:
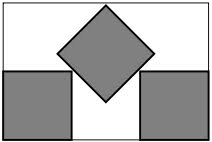 squares http://i39.tinypic.com/2vwvei0.jpg(source)
squares http://i39.tinypic.com/2vwvei0.jpg(source)
If
If I haven't made a mistake, you can take $\varepsilon$ to be as large as $1-\frac 23 \sqrt 2$.
- Does anybody know how to find good lower bounds on this number? Using the trick in the above picture, you can effectively get a layer of hypercubes whose length in a given direction is $\sqrt 2 -\frac 12$ instead of 1$1$. Is there a higher-dimensional version of this trick which does better?
- Does anybody know how to get good upper bounds on this number? In particular, is there an easy way to see that it's never possible to get $\prod_i a_i -1$?
