I think the whole list of Gjiergji (and lightly more, see below) follows from Wythoffian operations. For the cube-octahedron family, for example, consider the following diagram: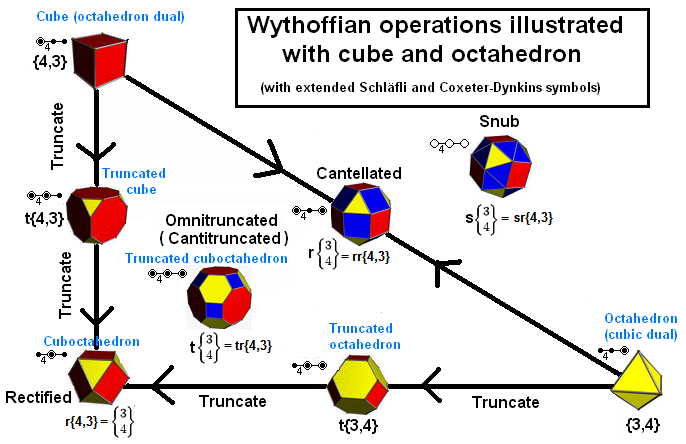

The polyhedron along an edge of the triangle is the Minkowski sum of the two vertices of that edge, which gives:

• The truncated cube is the Minkowski sum of a cube and a cube octahedron
• The truncated octahedron is the Minkowski sum of an octahedron and a cuboctahedron
• The rhombicuboctahedron is the Minkowski sum of an octahedron and a cube

The polyhedron inside the triangle is the Minkowski sum of the three vertices or, equivalently, the Minkowski sum of a vertex and the opposite edge. This gives:

• The truncated cuboctahedron is the Minkowski sum of a rhombicuboctahedron and a cuboctahedron

but also

• The truncated cuboctahedron is the Minkowski sum of a cube and a truncated octahedron.
• The truncated cuboctahedron is the Minkowski sum of an octahedron and a truncated cube.