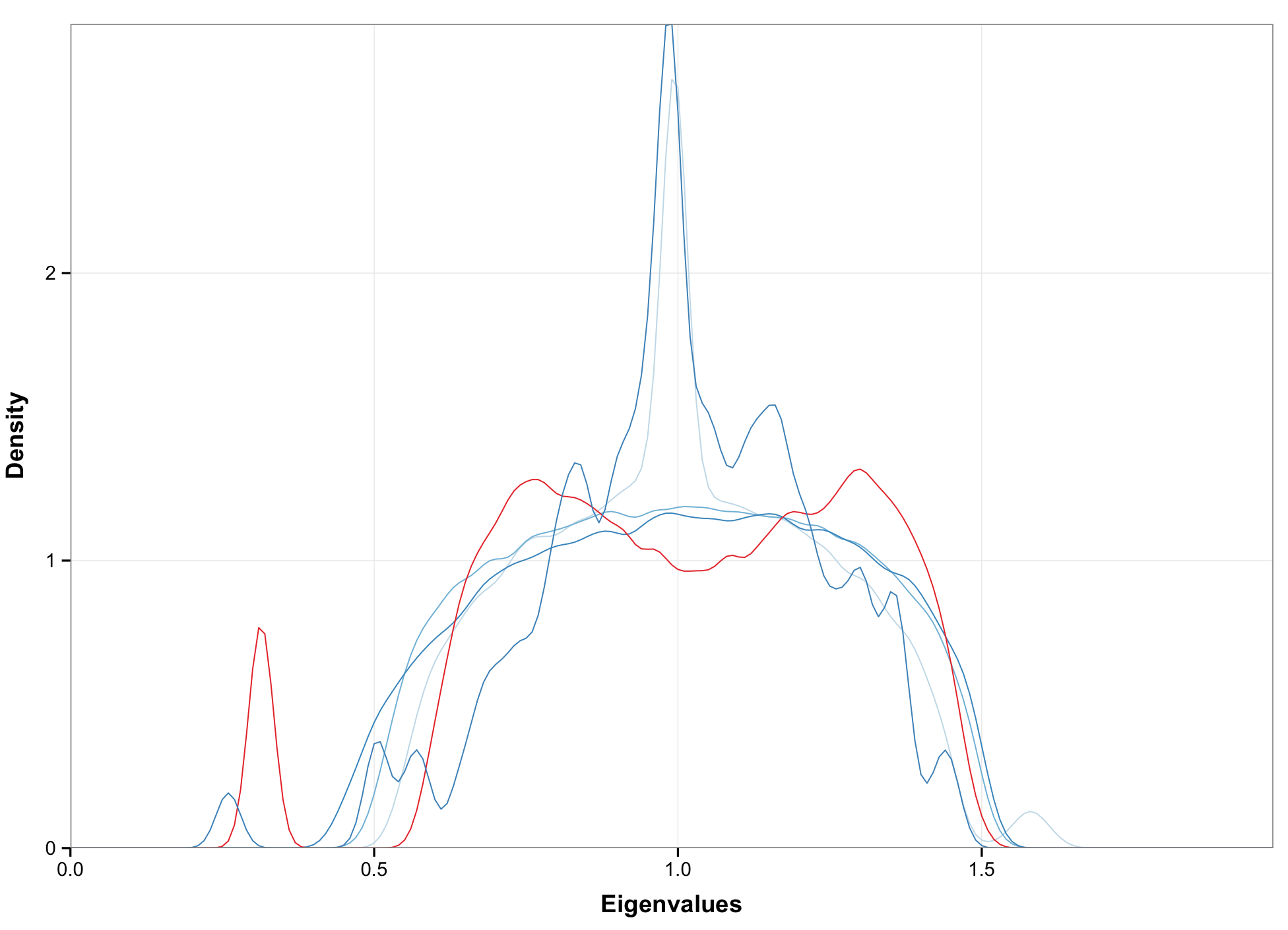
We often see normalized Laplacian spectra of graphs where density on eigenvalue 1 serves as an axis of symmetry, with particularly high (blue spectra in the figure) or low densities around this value (red spectrum in the figure) around this value.
- What does a high density on eigenvalue 1 (where 1 serves as an axis of symmetry) means in terms of graph structure (blue spectra)?
- Similarly, what does a low density on eigenvalue 1 (where 1 serves as an axis of symmetry, shaping a sort of rounded "M") means in terms of graph structure (red spectrum)?
- Is their a known class of graphs which follows the shape described in question 2 (red spectrum)?
Any literature on the subject is appreciated.