Let every point of $\mathbb{Z}^2$ be surrounded by a mirrored disk of radius $r < \frac{1}{2}$, except leave the origin $(0,0)$ unoccupied by a disk.
Q. Is it the case that every disk can be hit by a lightray emanating from the origin and reflecting off the mirrored disks?
Lightrays are composed of (infinitely thin) segments, and reflect off the disks
with angle of incidence equal to angle of reflection.
For example, here is one way (of many ways)
to hit the $(0,2)$ disk when $r = \frac{1}{4}$ with two reflections;
it clearly cannot be reached directly, with zero reflections:
PinballAll http://cs.smith.edu/~orourke/MathOverflow/PinballAll.jpg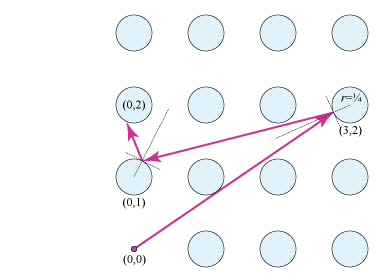
I believe the answer to my question Q is Yes, but I would be grateful for confirmation from the dynamical systems experts. (Forgive me if I have not learned sufficently from my previous, related question, "Pinball on the infinite plane.")
It occurs to me it might be interesting to color the disks according to the minimum number of reflections needed to hit each...
