The central construction feature of the surreal numbers is that it is Ord-saturated, which means that for any sets of surreal numbers $A$ and $B$, with $A<B$ in the sense that every element of $A$ is less than every element of $B$, then there is a surreal number $x$ that is above every element of $A$ and below every element of $B$. The first-born such number $x$ is denoted $$x=\{A\mid B\}.$$
In particular, when $B$ is empty, this means that $A$ is bounded above, and $\{A\mid\ \ \}$ is the first-born upper bound. Every set of surreal numbers admits an upper bound, but there is never a least upper bound, unless $A$ has a largest element.
This saturation fact implies that the surreal numbers are universal for every linear order. If you have a linear order $\langle L,\leq\rangle$, a hierarchy of your own devising, then there is an order preserving map from $L$ into the surreal numbers. One simply carries out a transfinite analogue of Cantor's back-and-forth construction (but one needs only forth for this universality). Namely, by the axiom of choice enumerate the elements of $L$ as $p_\alpha$ for some $\alpha<\kappa=|L|$, and then define $p_\alpha\mapsto x_\alpha$ in the surreals recursively. At stage $\alpha$, let $L_\alpha=\{x_\beta\mid \beta<\alpha\text{ and }p_\beta\leq p_\alpha\}$, and $R_\alpha=\{x_\xi\mid \xi<\alpha\text{ and }p_\alpha<p_\xi\}$, and then map
$$p_\alpha\mapsto x_\alpha=_{\text{def}}\{L_\alpha\mid R_\alpha\}.$$
This construction is order-preserving at every stage, and thus embeds $L$ into the surreals.
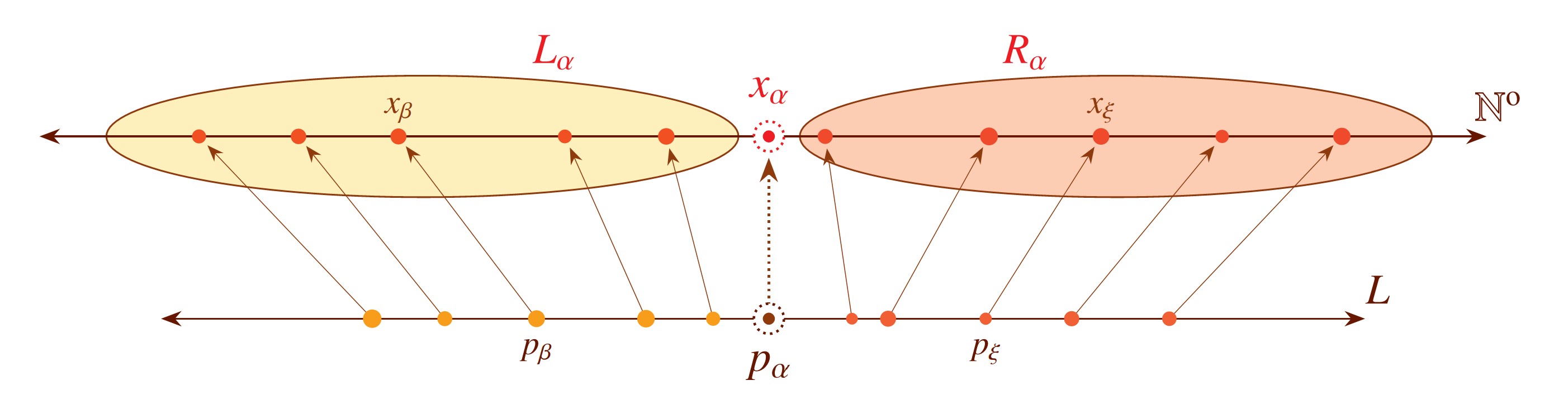 (The figure is from my essay on The Surreal numbers.)
(The figure is from my essay on The Surreal numbers.)
A modification of the argument enables one to preserve also the field structure, if indeed $L$ is a field, since one can ensure that $x_\alpha$ realizes the same type as $p_\alpha$ over the prior elements. So the surreals are universal for all ordered fields, including the Hardy fields.
One can handle class-sized orders and class-sized fields similarly, achieving universality for proper class structures, provided that the global choice axiom holds.
Since there are only continuum many functions on the reals, it seems to me that there are only a set of Hardy fields up to isomorphism, and so there are surreal numbers such that one can find suitable copies of any Hardy field below them.
But meanwhile, it seems to me that a given Hardy field can often have multiple copies in the surreals, as high as desired in the surreals, and so there will be no bound in the surreals that is above all possible copies of a Hardy field. The previous paragraph only bounds them up to isomorphism and not absolutely.

