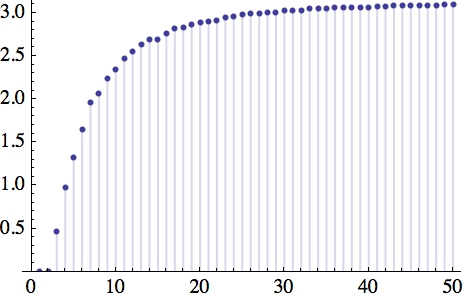
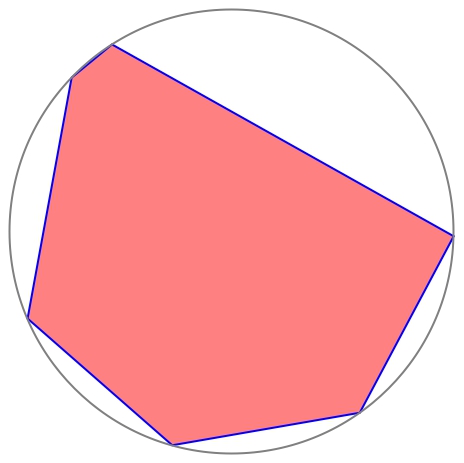
As mentioned in the comments, this question has been answered for random pointes on the boundary of convex bodies and even better for all intrinsic volumes. Let me offer some references:
A good refernce is:
Matthias Reitzner, Random points on the boundary of smooth convex bodies, Trans. Amer. Math. Soc. 354, 2243-2278, 2002
Abstract:
The convex hull of $n$ independent random points chosen on the boundary of a convex body $K \subset \mathbb{R}^d$ according to a given density function is a random polytope. The expectation of its $i$-th intrinsic volume for $i=1, \dots, d$ is investigated. In the case that the boundary of $K$ is sufficiently smooth, asymptotic expansions for these expected intrinsic volumes as $n \to \infty$ are derived.
By Ross M. Richardson, Van H. Vu and Lei Wu there are two papers, which are very simlilar:
Random inscribing polytopes, European Journal of Combinatorics. Volume 28, Issue 8, Pages 2057–2071, November 2007
and
An Inscribing Model for Random Polytopes, Discrete & Computational Geometry, Volume 39, Issue 1-3, pp 469-499, March 2008
With the following abstract:
For convex bodies $K$ with $\mathcal{C}^2$ boundary in $\mathbb{R}^d$ , we explore random polytopes with vertices chosen along the boundary of $K$. In particular, we determine asymptotic properties of the volume of these random polytopes. We provide results concerning the variance and higher moments of this functional, as well as an analogous central limit theorem.
Another more recent reference is
Károly J. Böröczky, Ferenc Fodor, Daniel Hug, Intrinsic volumes of random polytopes with vertices on the boundary of a convex body, Trans. Amer. Math. Soc. 365, 785-809, 2013, arxiv link
Let $K$ be a convex body in $\mathbb{R}^d$, let $j\in\{1, ..., d-1\}$, and let
$\varrho$ be a positive and continuous probability density function with
respect to the $(d-1)$-dimensional Hausdorff measure on the boundary $\partial
K$ of $K$. Denote by $K_n$ the convex hull of $n$ points chosen randomly and
independently from $\partial K$ according to the probability distribution
determined by $\varrho$. For the case when $\partial K$ is a $C^2$ submanifold
of $\mathbb{R}^d$ with everywhere positive Gauss curvature, M. Reitzner proved an
asymptotic formula for the expectation of the difference of the $j$th intrinsic
volumes of $K$ and $K_n$, as $n\to\infty$. In this article, we extend this
result to the case when the only condition on $K$ is that a ball rolls freely
in $K$.