Consider the following interesting theorem (7.5.7, p.294 in Topology and Groupoids by Ronald Brown):

Gluing theorem for adjunction spaces: Suppose that we have the following commutative diagram of topological spaces and continuous maps:

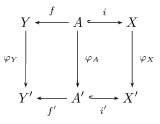
where $\varphi_{A}$, $\varphi_{X}$ and $\varphi_{Y}$ are homotopy equivalences, and the inclusions $i$ and $i'$ are closed cofibrations. Then the map

$$ \varphi:X\cup_{f}Y\rightarrow X'\cup_{f\phantom{l}'}Y' $$

induced by $\varphi_{A}$, $\varphi_{X}$ and $\varphi_{Y}$ is a homotopy equivalence.

In this post I asked a question that would become a corollary if the gluing theorem were true for arbitrary cofibrations $i$ and $i'$. I would like to know if this is the case. Otherwise, does anybody know of a counterexample?

Perhaps, it is possible that although the map $\varphi$ may not in general be a homotopy equivalence when the cofibrations $i$ and $i'$ are arbitrary, the adjunction spaces $Y\cup_{f}X$ and $Y'\cup_{f'}X'$ will nonetheless be homotopy equivalent. A possible approach to this would be to try to find a third space containing both as deformation retracts.

Note on notation: That an inclusion map $j:B\rightarrow Z$ is a closed cofibration means that it is a cofibration (i.e., the pair $(B,Z)$ has the homotopy extension property) and the set $B$ is closed in $X'$.

Thank you