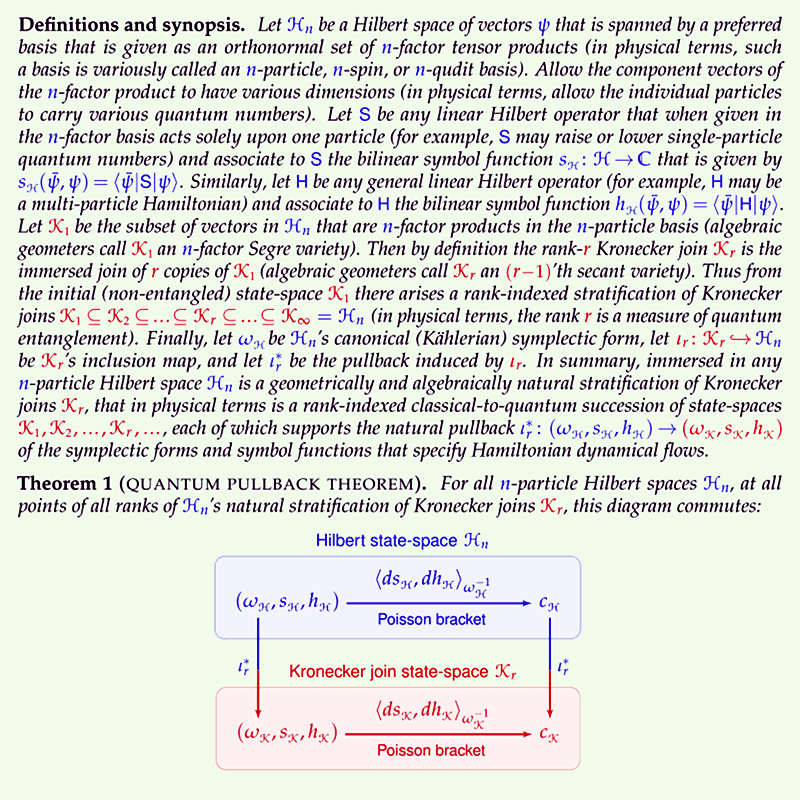
The preceding "toy models" focus mainly on algebraic properties of quantum state-spaces. Students who prefer a more geometric and/or PDE perspective on quantum dynamics may find it instructive to consider classical dynamical systems, which (historically) have always been a rich source of quantum dynamical "toy models".
Classical: The state-spaces of classical dynamical systems typically are endowed with both a symplectic structure and a metric structure. Canonical examples ("toy problems") include the Bloch equations and the Landau-Lifshitz-Gilbert equations: both have $S^2$ as their state-space; the symplectic and metric structures are natural; and in both cases dynamical trajectories unravel according to canonical fluctuation-dissipation relations.
Quantum: $C^n$ is the state-space; the symplectic and metric structures are natural (that is, Kählerian); and dynamical trajectories unravel according to canonical fluctuation-dissipation (that is, Lindbladian) relations.
In this geometric dynamical framework, what are often regarded as "spooky mysteries" of quantum mechanics are seen to be similarly present in classical theories; the main change is that global spectral theorems that apply in $C^n$ appear classically as local theorems involving dynamical flow.
These ideas are scattered throughout in the literature of math and physics, but there is at present no single textbook that develops this unified classical/quantum dynamical point of view. This for me defines " a book that you would like to write" (in Gil Kalai's phrase); further references to the literature can be found in that post.
In the meantime, a good "toy" exercise is to write down the symplectic and metric structures, and the Hamiltonian and Lindbladian potentials, that describe the Bloch equations in geometrically natural terms. In particular, an illuminating exercise is to derive via classical geometric methods the results that Charles Slichter's Principles of magnetic resonance derives quantum mechanically in Section 3.2, equation 3.7.
Note added: At the 52nd ENC Conference at Asilomar conference, we reduced the above ideas to practice, by redrafting Chapter III of Slichter's Principles of magnetic resonance in the language of geometric dynamics (PDF here, Wayback Machine). Here is the main theorem:
In essence, it turns out to be surprisingly easy to construct nonlinear quantum state-spaces that respect both thermodynamic "goodness" (that is, Kählerian structure) and quantum "goodness" (that is, Lindbladian structure).
Ought we to regard Kronecker state-spaces as legitimate quantum foil theories? Or are they merely useful computational idioms? Don't ask us ... as quantum simulations become more-and-more realistic, the division between quantum foil theories and practical computational tools is becoming less distinct.