$SU(1,1, \mathbb{C}) \simeq SL(2, \mathbb{R})$ as they preserve similar quadratic forms
* $|x|^2 -| y|^2=1$
* $ad-bc=1$
* let $x+y= a+bi$ and $x-y= c+di$
if instead of $ \mathbb{R}$ try a ring of integers $\mathcal{O}_K$ these are Bianchi groups. I am not am expert. the case of $\mathcal{O}_K=\mathbb{Z}[i]$ has explicit generators as found by Hurwitz
* the general case looks wide open. here is paper of [swan](http://www.sciencedirect.com/science/article/pii/0001870871900272)
* Bianchi original notes (in Italian) can be found on [Wikipedia](https://en.m.wikipedia.org/wiki/Bianchi_group) and make for interesting reading. [[1](https://eudml.org/doc/157598)]
* the [ Ford circles ](https://en.m.wikipedia.org/wiki/Ford_circle) and [Farey fractions](https://en.m.wikipedia.org/wiki/Farey_sequence) are relevant objects.
* if you google "Ford fractions" Ford's original discussion comes up. at the very end he briefly discusses $\mathbb{Z}[i]$ using spheres of various fractional radii. And the action of the Bianchi group.
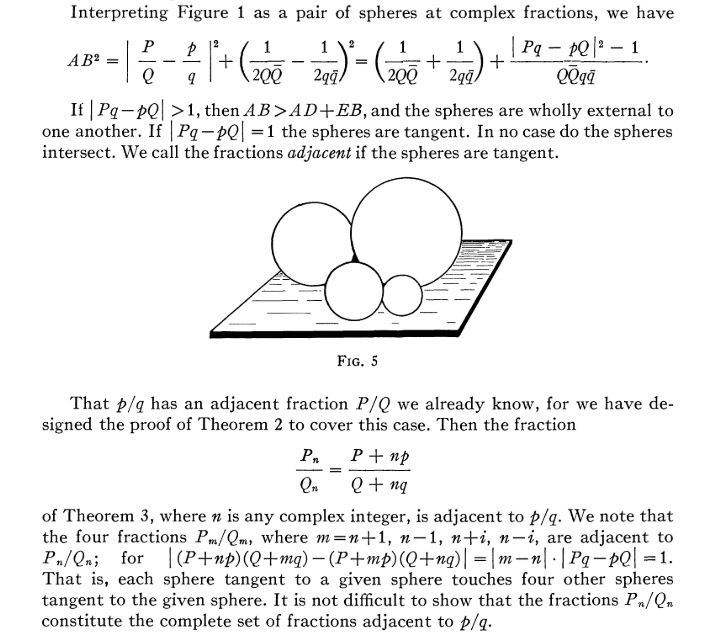
I find it utterly mind-boggling there is no easy extension of Farey fractions to general quadratic rings $\mathcal{O}_K$ with $K=\mathbb{Q}(\sqrt{-D})$. It's non-trivial