$SL_2(\mathbb{N})$ is a free monoid on the generators $$ L=\left(\begin{array}{cc}1&0\\1&1\\\end{array}\right),\quad R=\left(\begin{array}{cc}1&1\\0&1\\\end{array}\right). $$ Let $SL_2^{(n)}(\mathbb{N})$ designate the set of words of length $n$ in these generators. I have a couple of questions about this family that seem within reach (i.e. are already known or could be determined by the good folks of mathoverflow).

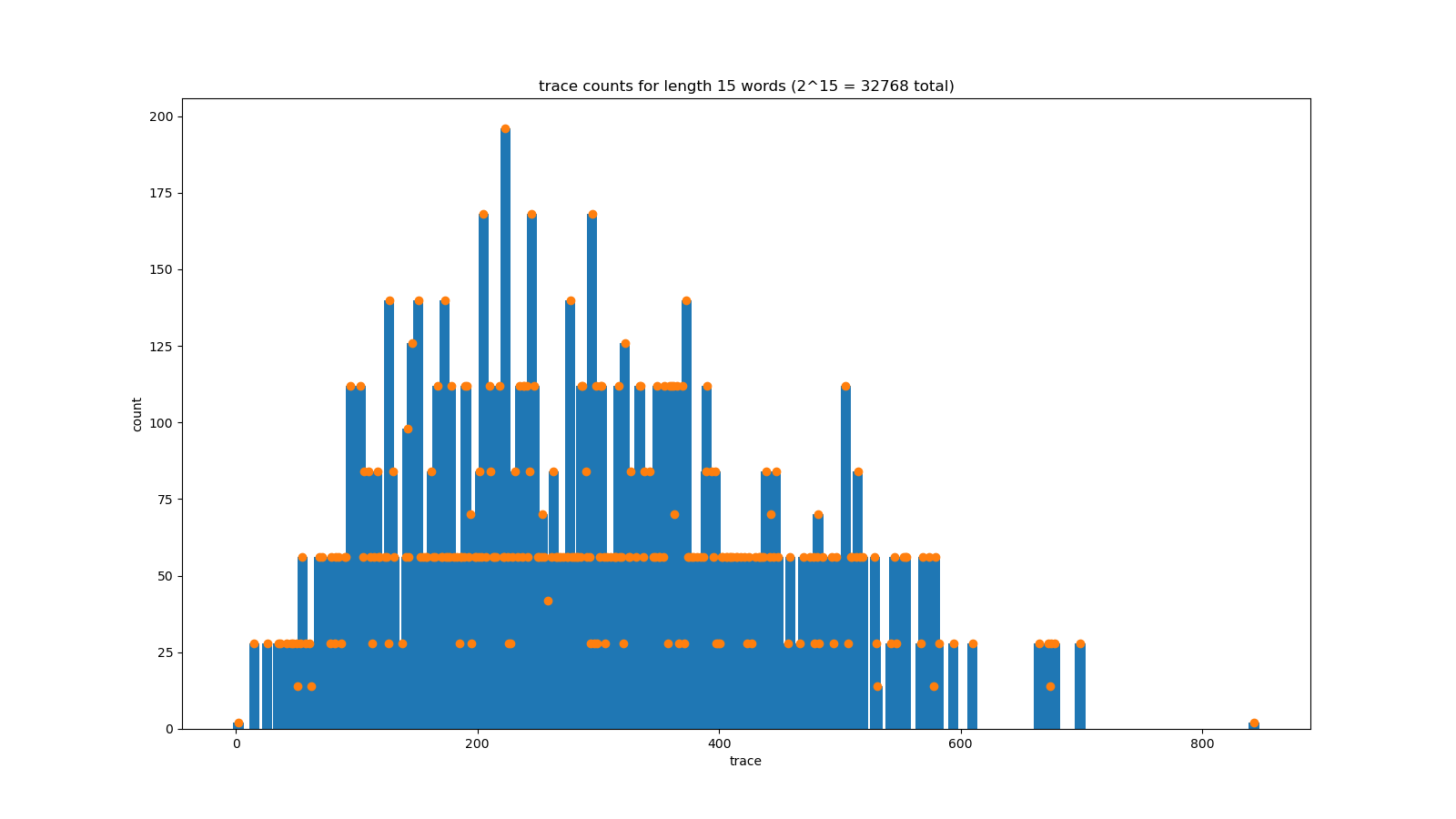
1. What is the distribution of $\{\mathsf{tr}(M) : M\in SL_2^{(n)}(\mathbb{N})\}$?
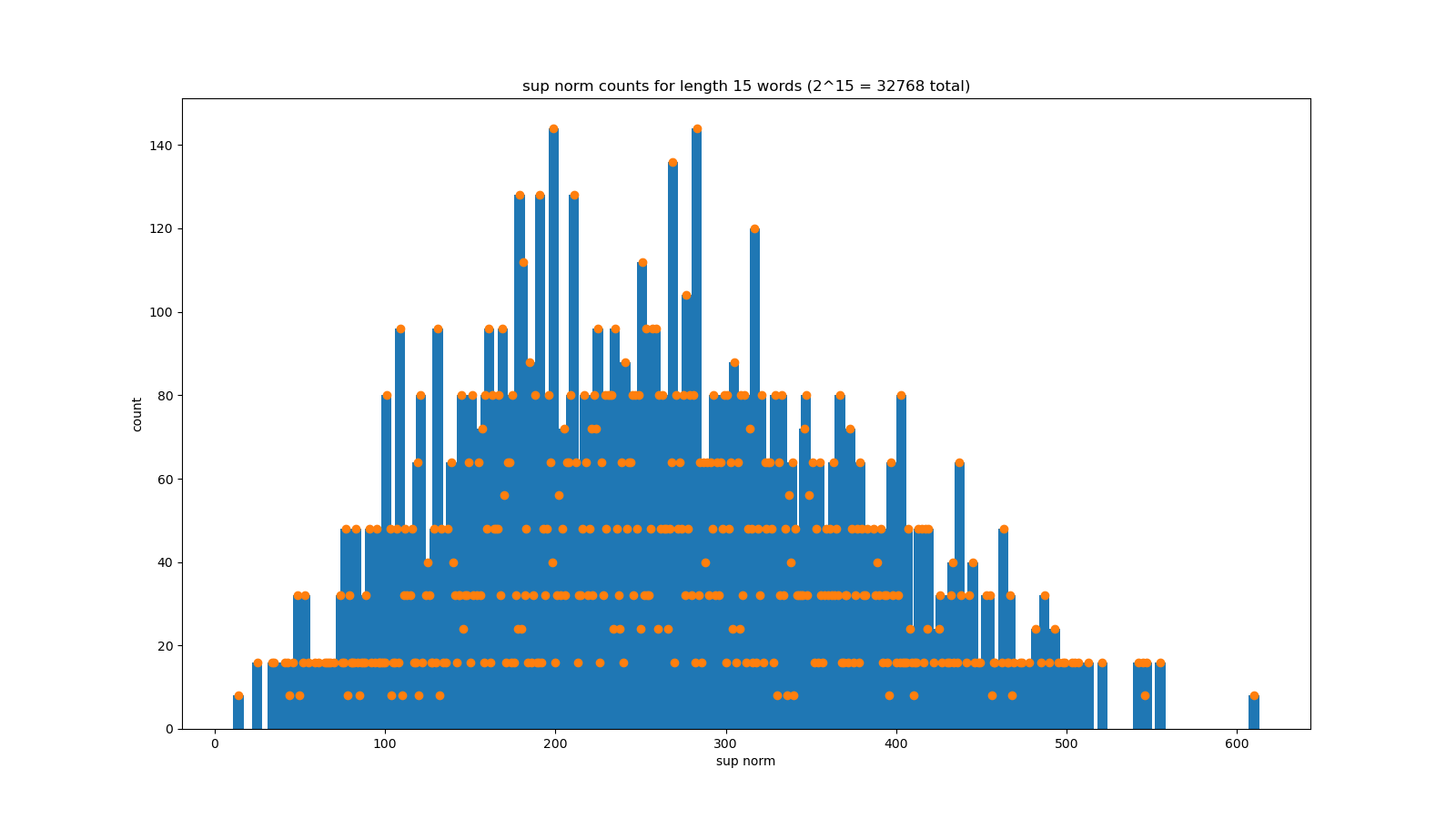
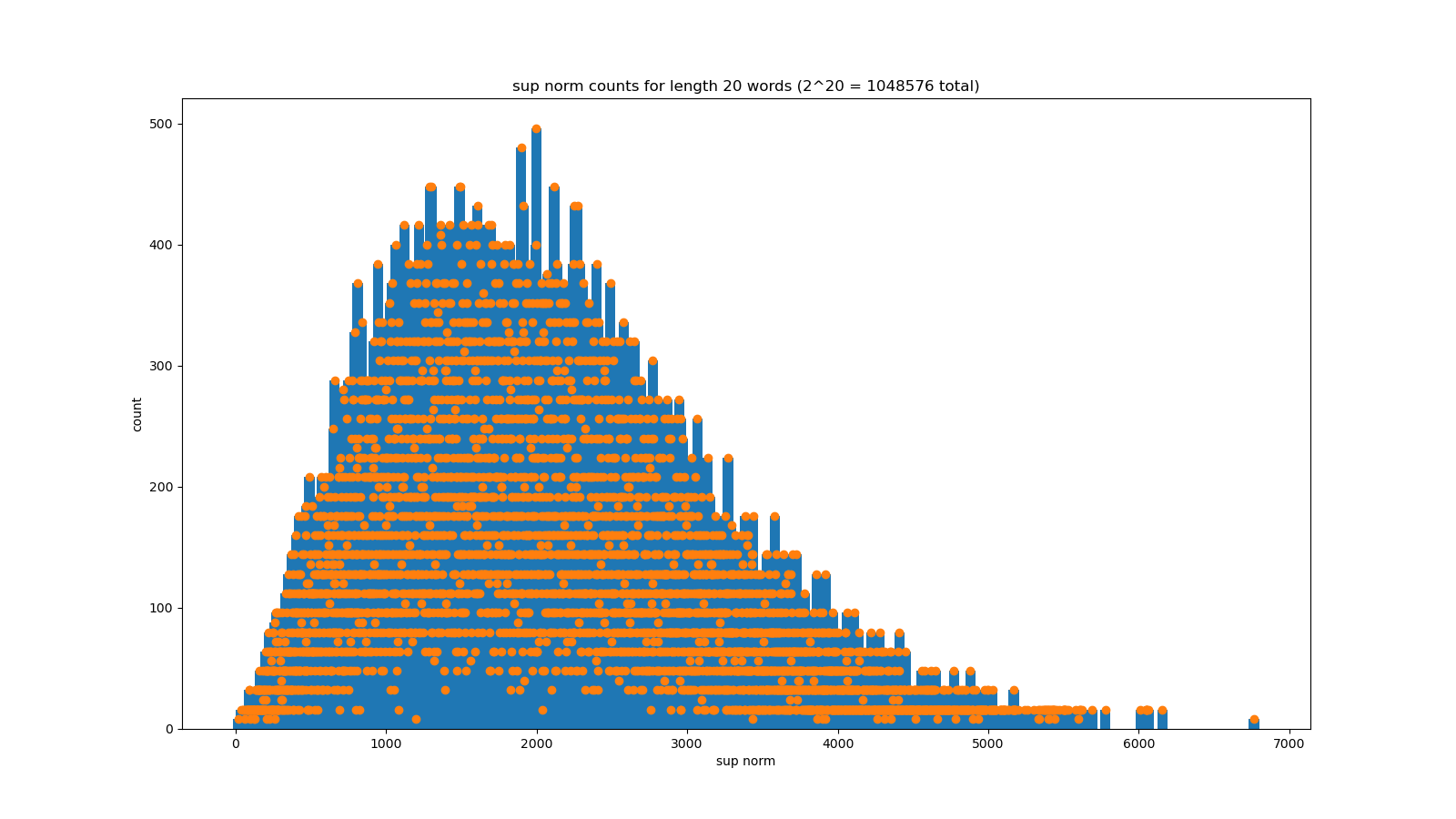
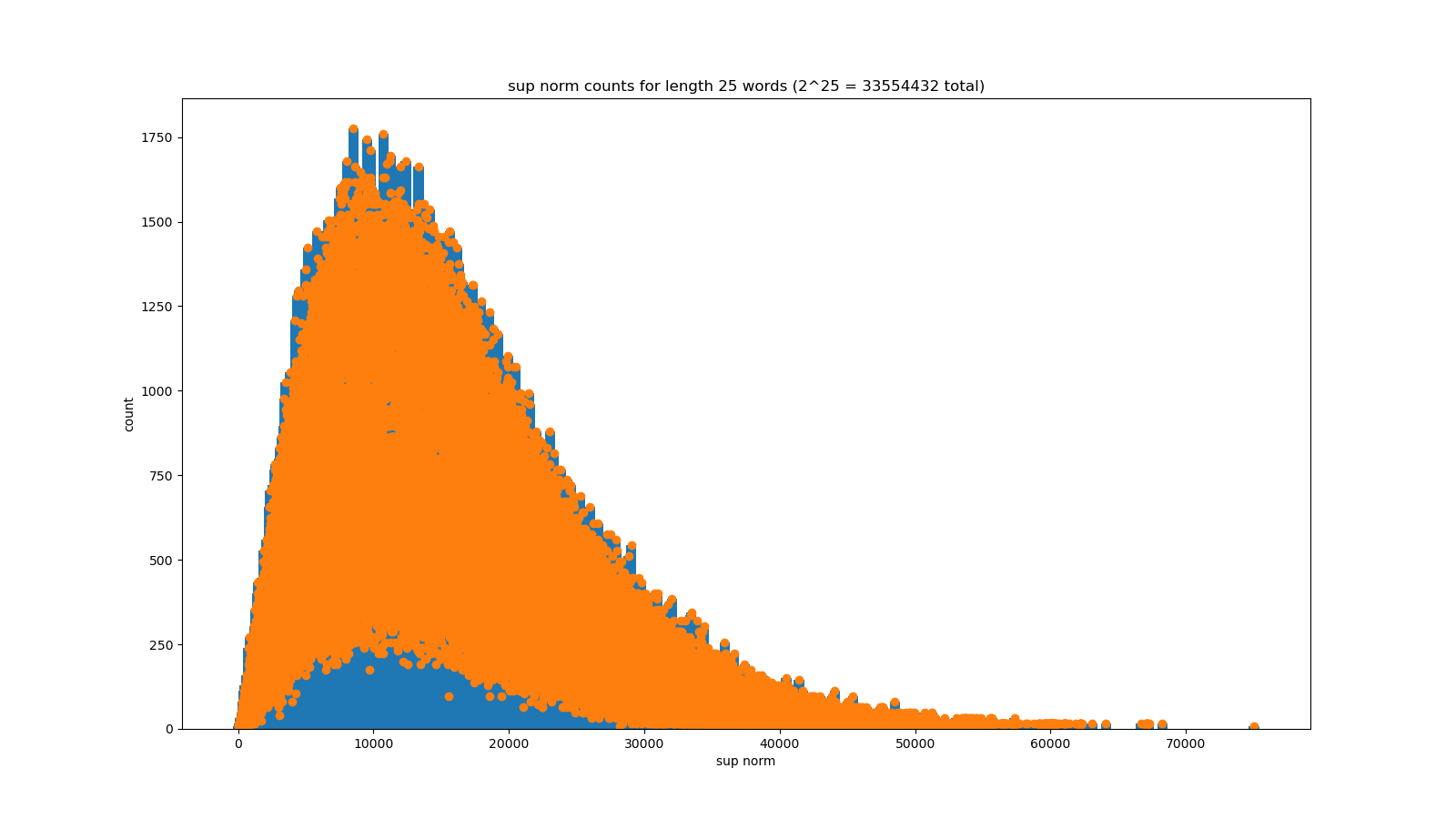
2. What is the distribution of $\{\|M\|_{\infty} : M\in SL_2^{(n)}(\mathbb{N})\}$?
3. What is the joint distribution of the two quantities above?

I'd prefer to know the limiting distribution $n\to\infty$ and some useful error term (if they exist), but any non-trivial bounds are welcome, or approximations to relevant functions of the matrices as functions of the $\{L,R\}$ decomposition $$ M(w)=\prod_{i=0}^{n-1}G_{w_i}, \quad w\in\{0,1\}^n, \quad G_0=L, \quad G_1=R, $$

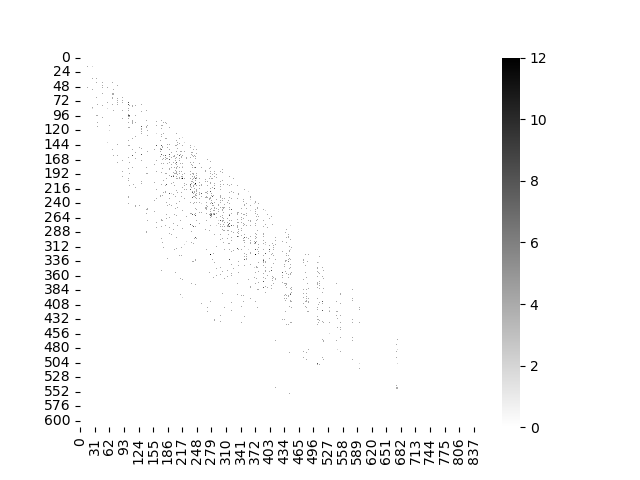
Here are a few pictures of (dubious quality); scatterplot on top of bar plot (could've just done a histogram but was more curious about some of the exact values).

Traces, $n=15,20,25$:

$L^{\infty}$, $n=15,20,25$:

Joint $n=15$ (the linear relationship seems to be $\mathsf{tr}(M)\approx \frac{9}{8}\|M\|_{\infty}$):