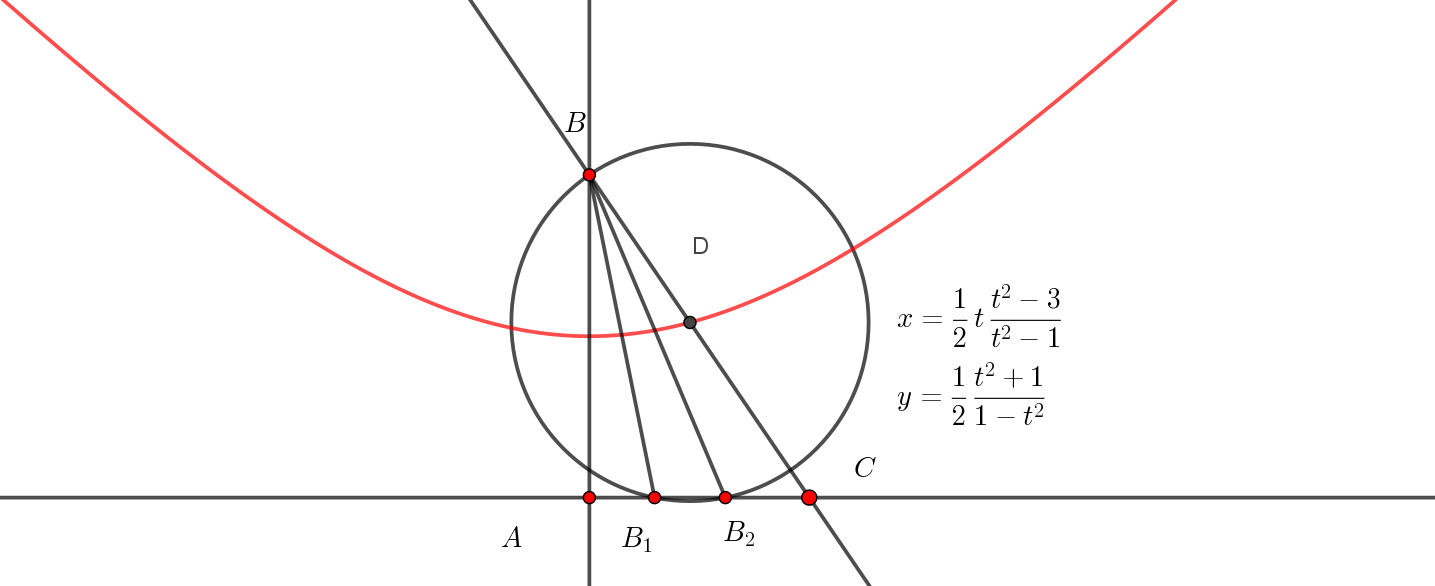
- Locus equation of the point $O_1$ as follows: In Cartesian coordinates, let curve with equation: $$x=\frac{1}{2}t\frac{t^2-3}{t^2-1}$$ $$y=\frac{1}{2}\frac{t^2+1}{1-t^2}$$ where $-1<t<1$ or the equation: $$x^2-y^2=\frac{2y^2-1}{2y+1}$$ where $y>0$.
- Let $ABC$ be a triangle with $A=(0,0), B(0,1)$ and $C$ lie on $Ox$, let $BC$ meets the curve at $D$, the circle circle $(D, DB)$ meets $AC$ at $B_1, B_2$ then $$\angle ABB_1=\angle B_1BB_1 = \angle B_2BC = \frac{\angle ABC}{3} $$
- Continuing what is the locus equation of $O_2, O_3, O_4,....$?