A finite, non-empty poset $P$ has dimension $k\in\mathbb N_0$ if $k$ is the smallest number of chains (totally ordered sets) into a product of which one can order-embed $P$.
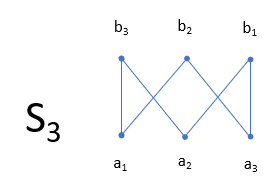
$S_3$ is the standard example; it has dimension $3$.
See W. T. Trotter, "The dimension of the cartesian product of partial orders," Discrete Math. 53 (1985), 255-263.
https://trotter.math.gatech.edu/papers/49.pdf
Also see George Bergman, "Some frustrating questions on dimensions of products of posets" (2024), preprint.