Consider some manifold $M$ say compact smooth. Let $b_i$ be its Betti numbers (non-zero), i.e. its cohomology dimensions.
Assume $M$ can be subsequently fibered by many manifolds, i.e. there is $ M_{i-1} \to M_{i} \to B_i$, for $i=1...N$ , $N$ is large, $M = M_N$. (For example $SU(N)$ is fibered like that: $SU(N-1) \to SU(N) \to S^{2N-1}$). So we can think of $M$ as kind of semi-direct product of $B_i$.
Question: Is it true that Betti numbers $b_i$ of $M$ can be well approximated by the Gaussian normal distribution under the conditions above ? (And say $N$ is much large than dimensions if $B_i$)
Motivation: If the fibration would be trivial one: $ M = B_1 \times B_2 \times ... \times B_N $, then it is true in rather simple way - Poincare polynom of the product is product of the Poincare polynoms, product of polynoms - is convolution if $Z\subset R$ so we are more or less in the setup of the classical central limit theorem and coefficients of the polynomial would be well approximated by the Gaussian.
Analogy: Non-triviality of fibration - is analogue of DEPENDENCE of the random variables in the central limit theorem.
Clearly small dependence of random variables would not affect much the central limit theorem, may be just slowing down the convergence - so we would need more variables to sum up - to get closer to Gaussian.
So the question is about the similar effect it topology - we have fibrations - so kind of dependence - but still we may hope to get the Gaussian if there are many many fibers .
Example:
Here are some examples of the approximation for $SU(N)$ (well, it is too trivial example since cohomologically $SU(N)$ IS direct product of $S^i$ i.e. Poincare polynom is direct product, but any way). Right picture is in log-scale, left is direct:
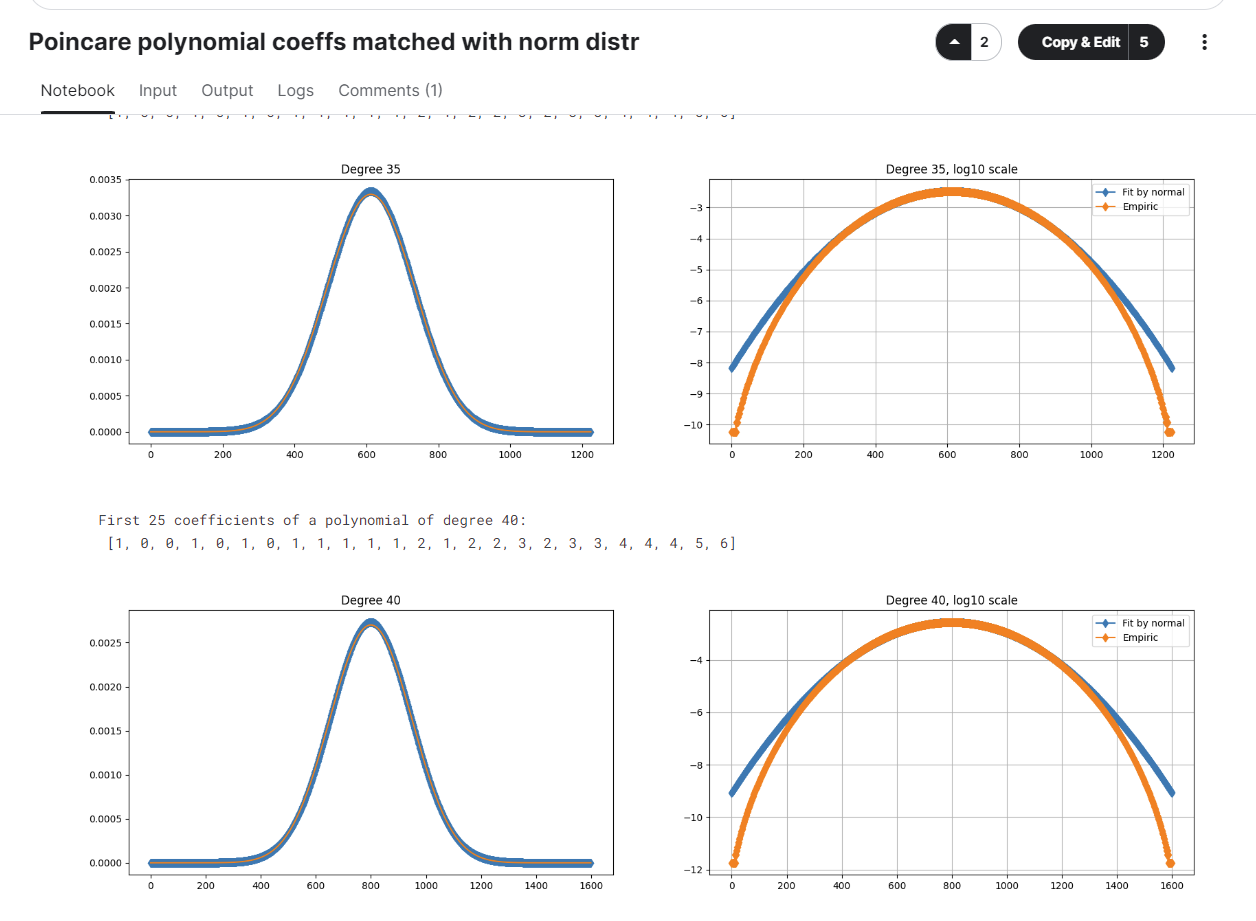 Thanks for EUGENE DURYMANOV for analysis:
https://www.kaggle.com/code/eugenedurymanov/poincare-polynomial-coeffs-matched-with-norm-distr
Thanks for EUGENE DURYMANOV for analysis:
https://www.kaggle.com/code/eugenedurymanov/poincare-polynomial-coeffs-matched-with-norm-distr
Group theory analogy
The group-theoretic counterpart of the question is discussed here: Growth of spheres in FINITE nilpotent groups - Gaussian approximation (central limit theorem)? I.e. growth of finite nilpotent groups might be well approximated by Guassian in some cases - based on the similar "fibration" idea: the role of the fibration is played by the central series of the group.
No-go for toric manifolds Another possible generalization - may seem to be toric manifolds - since torus - is direct product of $C^*$ - topologically like $S^1$ - so clearly Gaussian, and toric manifold is kind of close to it. But no : the first example of $CP^n$ with uniform Betti numbers shows it is not the case.
Full flags - it works - why ?
Another related story - full flag manifolds - Betti numbers - managed by $S_n$ (or other Weyl group Cayley graphs) and clearly follow the Gaussian distribution (e.g. follows from product formula or see: Growth functions of finite group - computation, typical behaviour, surveys?, Metrics on finite groups and generalizations of central limit theorems for balls volumes (à la Diaconis-Graham) , and see also https://mathoverflow.net/a/61490/10446 ).
Question2: Is there any topological fibration-like explanation of the Gaussian for full flag manifolds ?
