Let $G=\mathbb{Z}/\mathbb{2Z}$ act by the map $z\mapsto -z$ on a complex line $\mathbb{C}$. The category $\mathcal{Perv}(\mathbb{C})$ of perverse sheaves smooth along the stratification by the origin and its complement is equivalent to the category of diagrams of the form

$$\Psi\substack{\substack{u\\\longrightarrow}\\\substack{\longleftarrow\\v}}\Phi,$$ where $1-uv$ and $1-vu$ are invertible maps of finite-dimensional vector spaces. What is the category of equivariant perverse sheaves $\mathcal{Perv}_G(\mathbb{C})$?

Let's first think about the case of local systems on the open stratum. The group action takes a local system $L$ to an isomorphic local system $L'$, which can be identified with their stalks at 1 and -1 respectively with an automorphism $t$ corresponding to going around a counterclockwise loop, and these stalks may be identified by an isomorphism given by going around the, say, counterlockwise arc from 1 to -1. Then the axioms of an equivariant sheaves give us the description of an equivariant local system as a local system $L$ with an automorphism $\alpha$ such that $\alpha^2=t$.

Now, I can think microlocally about $\Psi$ and $\Phi$ as some microstalks on a punctured disk in the cotangent bundle, and then It seems to me that the category $\mathcal{Perv}_G(D)$ should be the category of diagrams

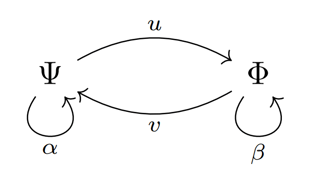
where $1-uv=t$, $1-vu=t$, $\beta^2=t$, $\alpha^2=t$, and it's hopefully clear enough without new notation to distinguish the monodromies $t$ on the two spaces.

On the other hand, I can also think of a perverse sheaves in terms of gluing data consisting of a local system $L$ on the open stratum $U$, a vector space $V$ as a local system on the closed stratum $Z$, and a pair of morphisms $\Psi(L)\to V\to \Psi(L).$ Then the group action should act trivially on $V$ and we arrive at a similar answer, except now $\beta^2=1.$

Which (if any) of the answers is correct? I seem to be assuming that the group action doesn't affect the canonical and variation maps, both of which I honestly still don't really understand.

We may also notice that $\mathbb{C}/G\cong\mathbb{C}$. This leads to a much more general, but probably very difficult question: If we are given a stratified variety $X$ on which $G$ acts in a stratum preserving way and the geometric quotient $X/G$ exists, what is the relationship between $\mathcal{Perv}_G(X)$, which is also the category of perverse sheaves on the quotient stack $(X/G)^{st},$ and $\mathcal{Perv}(X/G)$?