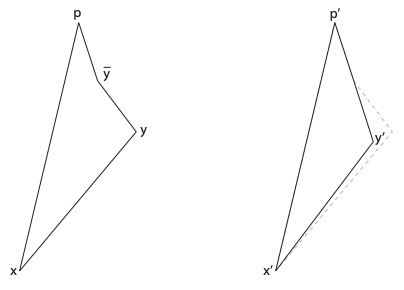
This is just a bit more elaboration on Anton's nice example, and subsequent comment. Here is the picture of the two triangles:
Note that $p'x'y'$ is obtained from $pxy$ by rotating the side $\overline{y}y$ about $\overline{y}$ until the interior angle at $\overline{y}$ reaches $\pi$. Since the angle at $y$ is obtuse, the points on the side $xy$ move closer to $p$. Thus we obtain $|p'z'|<|pz|$.
The quadrilateral $p\overline{y} y x$, which forms the space $X$, is CAT(0) because each of the subtriangles $p\overline{y}x$ and $\overline{y}yx$ is a convex subset of $\textbf{R}^2$ and a convex subset of a CAT(0) space is a CAT(0) space. Furthermore, gluing two CAT(0) spaces along isometric convex subsets (in this case the common edge $x\overline{y}$) yields a CAT(0) space. The quadrilateral $p\overline{y} y x$ may be regarded as a geodesic triangle $pxy$ in $X$.
Note that $X$ may be extended to a geodesically complete CAT(0) space, since we may regard $X$ as a geodesic triangle in a cone with vertex at $\overline{y}$. Setting the total angle of the cone at $\overline{y}$ to twice the interior angle of the quadrilateral at $\overline{y}$ makes sure that $py$ is indeed a geodesic. Smoothing the cone near $\overline{y}$ yields a Cartan-Hadamard manifold, i.e., a complete simply connected manifold of nonpositive curvature, where the comparison property in the question I had asked (and which holds in $\mathbf{H}^2$) does not hold.