This follows from a result of Hopf (see the exposition of Epstein). By this result, one may assume that there is a disk $D\subset N$ such that $f^{-1}(D)$ is $d$ disks mapping diffeomorphically to $D$, where $d$ is the degree of the map (we may assume $d$ is positive by switching orientation if needed).
Claim: Let $D\subset N$ be a ball. Then there is a map (homotopic to the identity) $h: N\to N$ such that $det(dh_x)=0$ for all $x\in N-D$ and $det(h_x)\geq 0$ for all $x$.
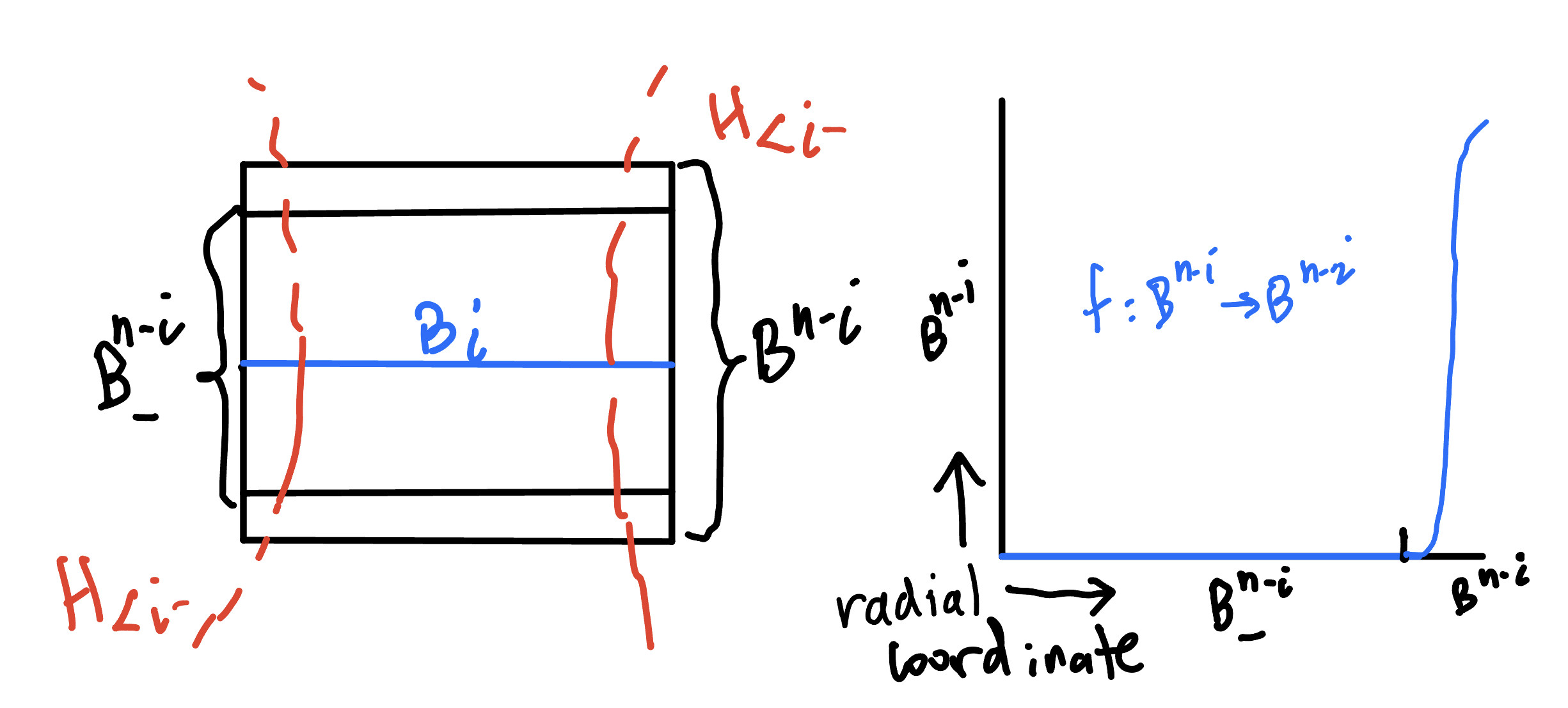
To prove this, cover $N$ with a smooth handle decomposition. This is a covering of $N$ by closed neighborhoods $H_0, \ldots, H_n$, $n=dim N$ (we assume the interiors cover $N$). Each $H_i$ is smoothly diffeomorphic to a disjoint union of $i$-handles, that is balls with a diffeomorphism to $B^i \times B^{n-i}$. Moreover, for each $i$-handle, $\partial B^i\times B^{n-i} \subset H_0\cup \cdots \cup H_{i-1}=H_{<i}$. The existence of a smooth handle decomposition follows eg from Morse theory. Moreover, we may assume that $H_n$ is a single $n$-handle which we may identify with our ball $D$ by a diffeomorphism.
Choose slightly smaller handle neighborhoods $H_{i-}$ with handles $B^i\times B_-^{n-i} \subset B^i\times B^{n-i}$ so that the interiors of these handles cover as well. We have smooth maps $g_i: N\to N$ so that ${g_i}_{|N-H_i}=Id$, $g_i(H_i)=H_i$, $det((dg_i)_x)=0$ for all $x\in B^i\times B_-^{n-i} - \cup_{j<i} H_{j-} $, ${g_i}_{|B^i\times B^{n-i}}$ preserves the $\{x\}\times B^{n-i}$ slices on the handle, $g_i$ sends $B^i \times B_-^{n-i}$ to $B^i\times \{0\}$ outside of $ \cup_{j<i} H_{j-} $ , and $det((dg_i)_x)\geq 0$ for all $x\in N$ *. Then the composition $h=g_{n-1}\circ \cdots \circ g_0$ will have $det(dh_x)\geq 0$ for all $x\in N$ and $det(dh_x)=0$ for $x\in N-D$ (since by construction $det(dh_x)=0$ for $x\in \cup_{i<n} H_{i-}$).
Then $h\circ f$ will have the desired property.
- The map $g_i$ restricted to each handle preserves the $\{x\}\times B^{n-i}$ directions on each handle. In the $B^{n-i}$ direction one uses a bump function (such as my favorite the Fabius function) to make a function $f:B^{n-i}\to B^{n-i}$ sending $B^{n-i}_-$ to $0$ (see the diagram for a picture in the radial coordinate). Then use a partition of unity in the $B^i$ radial coordinate so that in $H_{<i}$ near $\partial B^i\times B^{n-i}$ the map is the identity in the $B^{n-i}$ direction, and in $B^i\times B^{n-i}-H_{<i-}$ the map is $f$.