Your conjecture does not hold in general.
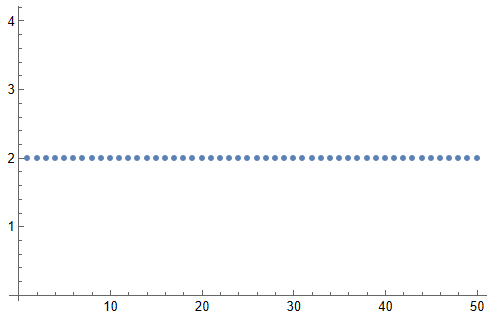
Indeed, for $m=5$ and all natural $n$ we have $$d_{m,n}:=d(J_m^{(n)}, \{i/m\}_{i=1}^{m-1}) =\frac2{mn}. \tag{1}\label{1}$$
To prove this, one can check that, letting $$M_{n,i}:=\min\{|i/5-y|\colon y \in J_5^{(n)}\},$$ we have
- if $n\equiv0$ or $n\equiv4$ ($\mod5$), then $M_{n,1}=\frac2{5n}$;
- if $n\equiv1$ ($\mod5$), then $M_{n,4}=\frac2{5n}$;
- if $n\equiv2$ ($\mod5$), then $M_{n,2}=\frac2{5n}$;
- if $n\equiv3$ ($\mod5$), then $M_{n,3}=\frac2{5n}$.
So, for all natural $n$ we have $d_{5,n}=\max_{0\le i\le4}M_{n,4}\ge\frac2{5n}$ (which already disproves the conjecture).
On the other hand, one of course has $d_{5,n}\le\frac2{5n}$ for all natural $n$. So, \eqref{1} holds for $m=5$ and all natural $n$. $\quad\Box$
To illustrate \eqref{1}, below is the graph $\{(n,m\, n\, d_{m,n})\colon n=1,\dots,50\}$ for $m=5$: