An identity $E$ that obeys all the claimed properties is $$ E: x+(y+z) = (x+y)+w \hbox{ for all } x,y,z,w.$$
- $E$ is implied by triple constancy (and hence by constancy): obvious since both sides are constant in this case
- $E$ does not imply triple constancy (and hence does not imply constancy either): follows from considering the left-zero semigroups $x+y=x$ mentioned by arsmath
- $E$ implies associativity: obvious by specializing to $w = z$
- $E$ is not implied by associativity: follows from considering (say) addition on the integers
This candidate $E$ was located by pursuing the analysis in Pace's answer to isolate the form that $E$ had to take as much as possible, as described in the comments to that answer. With a little more effort, it should be possible to entirely classify (up to relabelings and symmetries) the full set of identities $E$ that answer the question.
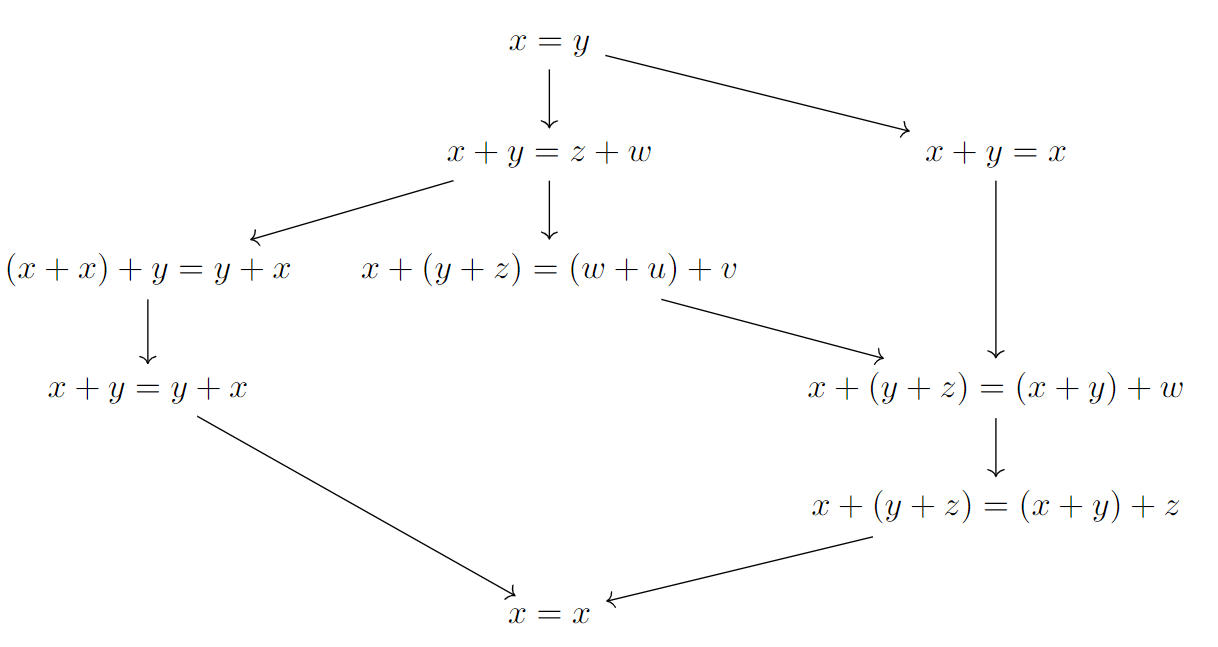
Here is the Hasse diagram of the various identities discussed on this page and on the related question linked by the OP, where the ordering is from stronger identities to weaker ones:
It might be a suitable undergraduate research project to extend this diagram to cover other short identities for magmas. EDIT: It might be a suitable graduate research project to find a way to do this automatically using proof assistants and possibly also machine learning/AI tools. (Some further discussion of this latter possibility can be found here, where I pose a concrete challenge of using such tools combined with human expert-hours to extend the above Hasse diagram to the five thousand or so other universal equational laws for magmas that involve at most four applications of the binary operation $+$.)