To complete the 12 cfracs in this post and the 4 in the next, all associated with 16 "sporadic sequences", then 13 of them have closed-forms, 1 has six limits (also with closed-forms but one divergent), and the last 2 as divergent.
We evaluate the last 3 cfracs for $n = 1000\; \text{to}\; 1200,$ sort the values $v$, and plot the values $-2<v<2.$
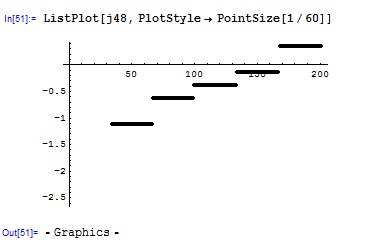
I. Degree 2 for (-9,-3,-27)
$$C_2(-9,-3,-27) = \frac1{-3 + \large{\underset{k=1}{\overset{n}{\mathrm K}} ~ \frac{-27k^4}{(-9k^2-9k-3)}}}$$
As discussed in the post above, this has six limits as clearly seen in the plot below (the divergent $v \to \infty$ is not shown),
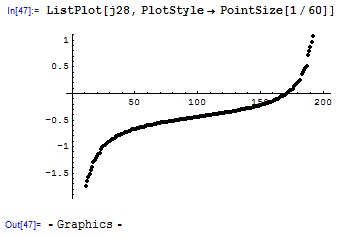
II. Degree 3 for (11,3,1)
$$C_3(11,3,1) = \frac1{-5 + \large{\underset{k=1}{\overset{n}{\mathrm K}} ~ \frac{-125k^4}{-(2k+1)(11k^2+11k+5)}}}$$
Its plot is vastly different and presumably has infinitely many limits within $-1<v<0$,
It has an illusory "pattern" if $n$ is mod $5$ or mod $7$, but disappears upon further analysis.
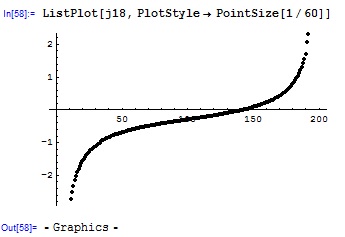
III. Degree 3 for (7,2,8)
$$C_3(7,2,8) = \frac1{-3 + \large{\underset{k=1}{\overset{n}{\mathrm K}} ~ \frac{-81k^4}{-(2k+1)(7k^2+7k+3)}}}$$
Likewise, its plot looks very similar to the previous one,
so the same conclusion about it can be made.