$\newcommand\R{\mathbb R}$Update: Within the previously established framework, we now show that it is enough to have just $N=4$ points. This improves what seems to be the previous record, of $N=5$. It should be clear that this new record cannot be further improved. Details on this are given at the end of the answer.
The answer is no.
Let us use the terms "acute angle" and "positive" in the nonstrict sense, meaning "angle $\le\pi/2$" and $\ge0$, respectively. The "strict" modification should be straightforward. By approximation, we may also assume that the set of vectors is allowed to be infinite.
Now, for any vector $u$ in $\R^d$ ($d\ge3$) with Euclidean norm $|u|=1$, consider the set
\begin{equation*}
S_u:=\{u+v\colon\, v\in\R^d,|v|=1,v\cdot u=0\}, \tag{10}\label{10}
\end{equation*}
with $\cdot$ denoting the dot product. (One may note that (say) for the $d=3$ the set $S_u$ is the unit-radius circle in $\R^d$ centered at $u$ and lying in the affine plane through $u$ perpendicular to $u$.)
Then for any vector $u+v\in S_u$ the angle between $u+v$ and $u$ is $\pi/4$ and hence, by the subadditivity of the angle measure, the angle between any two vectors in $S_u$ is acute. One can also show this algebraically: For any $u+v$ and $u+v'$ in $S_u$, we have $(u+v)\cdot(u+v')=1+v\cdot v'\ge0$, by the Cauchy--Schwarz inequality.
Moreover, any rotation of $S_u$ is of the same form, $S_u$, but maybe for another unit vector $u$.
It remains to show that $S_u\not\subseteq\R_+^d$ for any unit vector $u$. Take indeed any such vector $u=(u_1,\dots,u_d)$. Without loss of generality, $|u_1|$ is the smallest of all the $|u_j|$'s, so that $$|u_1|-\sqrt{1-u_1^2}<0.$$ Let now \begin{equation} v=v^* :=(v_1,\dots,v_d):=\Big(-\sqrt{1-u_1^2},\frac{u_1u_2}{\sqrt{1-u_1^2}},\dots,\frac{u_1u_d}{\sqrt{1-u_1^2}}\Big). \tag{15}\label{15} \end{equation} Then $u+v\in S_u$. However, $u_1+v_1<0$, so that $S_u\not\subseteq\R_+^d$. $\quad\Box$
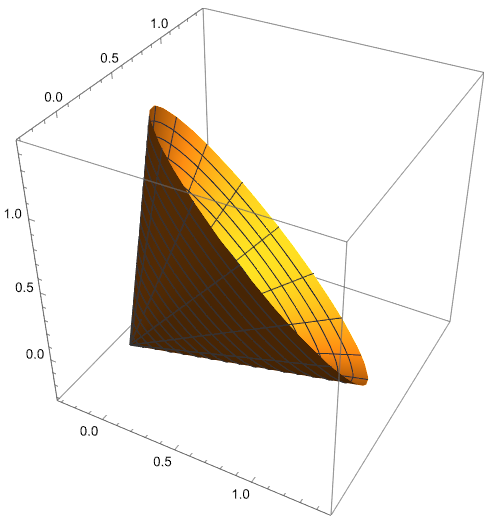
The picture below shows the set $[0,1]S_u=\{tw\,\colon t\in[0,1],w\in S_u\}$ for $d=3$ and (the apparently "best") unit vector $u=\frac1{\sqrt3}\,(1,1,1)$. It is kind of seen that $[0,1]S_u\not\subseteq\R_+^d$ and hence $S_u\not\subseteq\R_+^d$.
Added: Since the OP wanted an explicit finite set of vectors (rather than the infinite set $S_u$), let us provide such a set. So, for $d=3$, let $S_{u,N}$ be the set of all $N$ vertices of a regular $N$-gon inscribed into the circle $S_u$. Then the shortest distance from the point $v^*$ defined in \eqref{15} (and actually from any point on the circle $S_u$) to the set $S_{u,N}$ will be no greater than the length $2\sin\frac{2\pi}{4N}$ of a side of a regular $2N$-gon inscribed into the unit circle $S_u$. So, following lines of above reasoning, we see that, to get $w_1<0$ for at least one vertex $w=(w_1,\dots,w_3)$ of $S_{u,N}$, it is enough that $\frac1{\sqrt3}-\sqrt{1-(\frac1{\sqrt3})^2}+2\sin\frac{2\pi}{4N}<0$. The latter inequality holds for all natural $N\ge14$. Thus, we get a counterexample with a set of $14$ vectors. This is worse than $N=5$ in Nathaniel Johnston's answer; however, here the reasoning is quite elementary. (The number $N=14$ can apparently be improved even within this elementary framework, using a bit more complicated consideration.)
Details on the update: As in the paragraph just above, let $d=3$ and consider the set $S_{u,4}$, that is, the set $S_{u,N}$ with $N=4$, so that $S_{u,4}$ is the set of the vertices of a square inscribed into the circle $S_u$, defined in \eqref{10}. So, \begin{equation*} S_{u,4}=\{u+V_j\colon j\in\{0,1,2,3\}\}, \end{equation*} where \begin{equation*} V_j:=a\cos\Big(t+\frac{2\pi j}4\Big)+b\sin\Big(t+\frac{2\pi j}4\Big)=(V_{j,1},V_{j,2},V_{j,3})\in\R^3, \end{equation*} $t\in[0,\pi/2]$ is the angle of the rotation of the vertices of the square about the axis through the vector $u$, and $u,a,b$ are orthonormal vectors in $\R^3$. To be specific, let \begin{equation*} a=\Big(\sqrt{1-u_1^2},-\frac{u_1u_2}{\sqrt{1-u_1^2}},-\frac{u_1 \sqrt{1-u_1^2-u_2^2}}{\sqrt{1-u_1{}^2}}\Big) \end{equation*} and \begin{equation*} b=\Big(0,\frac{\sqrt{1-u_1^2-u_2^2}}{\sqrt{1-u_1^2}},-\frac {u_2}{\sqrt{1-u_1^2}}\Big), \end{equation*} with \begin{equation*} u=(u_1,u_2,u_3). \end{equation*} Here it is assumed that $u_1^2\ne1$, which can be done without loss of generality (wlog) -- otherwise, rearrange the coordinate axes.
We want to show that \begin{equation*} S_{u,4}\overset{\text{(?)}}{\not\subseteq}\R_+^3 \tag{20}\label{20} \end{equation*} for any described choices of $u$ and $t$.
Wlog, \begin{equation*} u_1\le u_2\le u_3. \end{equation*}
Suppose for a moment that $u_1\le0$. Clearly, $V_{j,1}<0$ for at least one $j\in\{0,1,2,3\}$, and then $u+V_j\in S_{u,4}\setminus\R_+^3$, so that \eqref{20} holds.
So, wlog \begin{equation*} 0<u_1\le u_2\le u_3=\sqrt{1-u_1^2-u_2^2}. \tag{30}\label{30} \end{equation*} Note that \begin{equation*} \begin{aligned} &\{u_1+V_{j,1}\colon j\in\{1,2\}\} =\left\{u_1-\sqrt{1-u_1^2}\,\sin t,u_1-\sqrt{1-u_1^2}\,\cos t\right\}. \end{aligned} \end{equation*} So, if \eqref{20} fails to hold, then \begin{equation*} u_1\ge \sqrt{1-u_1^2}\,\max(\sin t,\cos t)\ge\frac1{\sqrt2}\,\sqrt{1-u_1^2} \tag{40}\label{40} \end{equation*} and hence $u_1\ge\frac1{\sqrt3}$, which implies \begin{equation*} u_1=u_2=u_3=\frac1{\sqrt3}, \end{equation*} in view of \eqref{30}. Moreover, if we had $t\in[0,\pi/2]\setminus\{\pi/4\}$, then \eqref{40} would imply $u_1>\frac1{\sqrt3}$, which would contradict \eqref{30}.
So, if \eqref{20} fails to hold, then \begin{equation*} u_1=u_2=u_3=\frac1{\sqrt3}\quad\text{and}\quad t=\pi/4. \end{equation*} But then \begin{equation*} V_{0,3}=\frac{\sqrt{1-u_1^2-u_2^2} \left(\sqrt{1-u_1^2}-u_1 \cos t\right)-u_2 \sin t}{\sqrt{1-u_1^2}}=-0.211\ldots<0. \end{equation*} Thus, in all cases, if \eqref{20} fails to hold, then it necessarily holds. $\quad\Box$