As requested by the OP, I am making an answer from my comments to the question.
This is all standard material but I agree with him that it might be useful for somebody interested in this material.
Still, it might be better to migrate the whole stuff to math.SE. Please just vote for it if you think so.
Given any function $f$ on $\mathbb S^2$ with $\tau_A(f)=\lambda_Af$ for all $A\in\operatorname{SO}(3)$ as in the question, we will have$$f(Av)=\tau_{A^{-1}}(f)(v)=\lambda_{A^{-1}}f(v)$$for all $A$ and $v$, where we identify $\mathbb S^2$ with the set of all unit length vectors.
It follows that $f(ABv)=f(BAv)$ for all $A,B\in\operatorname{SO}(3)$ and all $v\in\mathbb S^2$.
This implies that $f$ is a constant function. Indeed given any unit vectors $v_1$, $v_2$, there is a $B\in\operatorname{SO}(3)$ with $v_2=Bv_1$. Let $v$ be a unit vector along the rotation axis of $B$, i. e. such that $Bv=v$; then there is an $A\in\operatorname{SO}(3)$ with $v_1=Av$. Then, $$ f(v_1)=f(Av)=f(ABv)=f(BAv)=f(Bv_1)=f(v_2). $$
This argument clearly depends on noncommutativity of $\operatorname{SO}(3)$, but not only on noncommutativity. Rather, declaring $AB=BA$ for all $A$, $B$ collapses $\operatorname{SO}(3)$ to the trivial group, i. e. $\operatorname{SO}(3)$ is a perfect group, which is stronger than just noncommutativity.
For example, the subgroup of $\operatorname{SO}(3)$ generated by all rotations around the $z$ axis together with the transformation sending $(x,y,z)$ to $(y,x,-z)$ is noncommutative — it is the (continually) infinite dihedral group — but it admits a nonconstant eigenfunction $f(x,y,z)=z$.
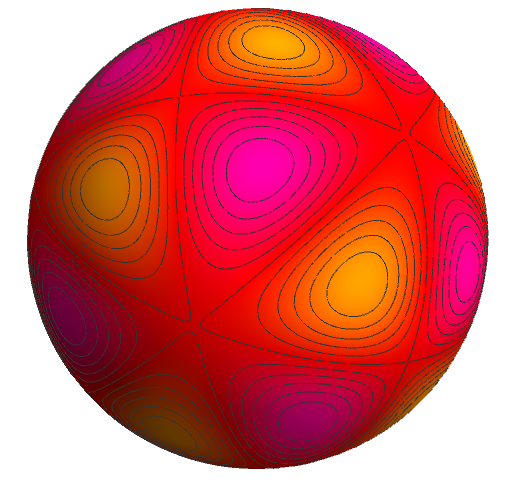
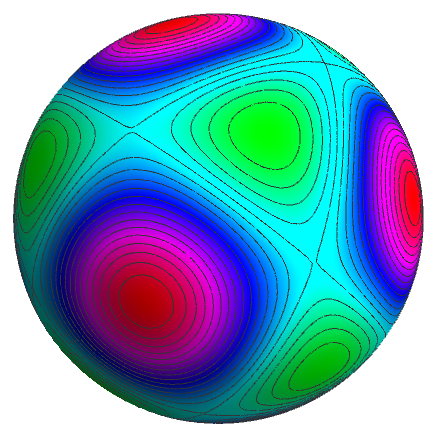
For another example, the group of rotations of the unit cube is a noncommutative subgroup of order $24$ in $\operatorname{SO}(3)$ which has common eigenfunctions $f(x,y,z)=xyz$, $(x^2-y^2)(x^2-z^2)(y^2-z^2)$, $x^4+y^4+z^4$.
Just for fun, here are the level lines of these functions on $\mathbb S^2$, clearly exhibiting cubical symmetry:
$xyz$
$(x^2-y^2)(x^2-z^2)(y^2-z^2)$
$x^4+y^4+z^4$