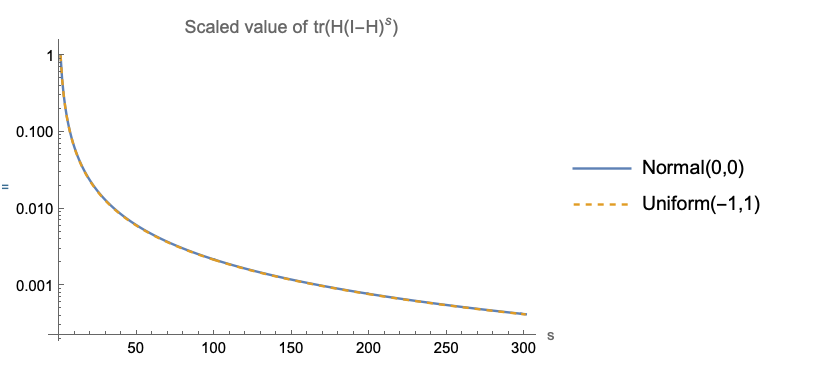
Suppose $H$ is a random positive definite matrix normalized to have norm 1. Can we say anything about behavior of $f(s)$?
$$f(s)=\operatorname{Tr}[H(I-H)^s]$$
Taking $H=A^T A$ with entries of $A$ sampled from standard normal or uniform(-1,1) produces essentially identical curves, which suggests universality. What is the shape of this curve?
Motivation: $f(s)$ gives the loss observed after $s$ steps of gradient descent on quadratic $H$ (math). I'm interested in understanding behavior of this decay so that I could extrapolate $f(t)$ from few observations of loss on an known quadratic $H$. Theoretically, eigenvalues of $H$ can be reconstructed from values of $f(t)$ by using inverse Laplace transform, but not numerically stable