The following is for $\epsilon\geq c\vee 1$ for some random constant. For less than $c$, it is unclear how to modify the argument below. Perhaps some scaling argument can do it.
Also, the following is proving a much stronger result, than what you ask because I tried to do with local time since fBM doesn't have any Markov property. So a weaker argument should work to give it for all $\epsilon$; any ideas are welcome.
The local time $\ell_{x,t}$ of fractional Brownian motio is continuous eg."A uniform result for the dimension of fractional Brownian motion level sets" or "On the local time of multifractional Brownian motion"
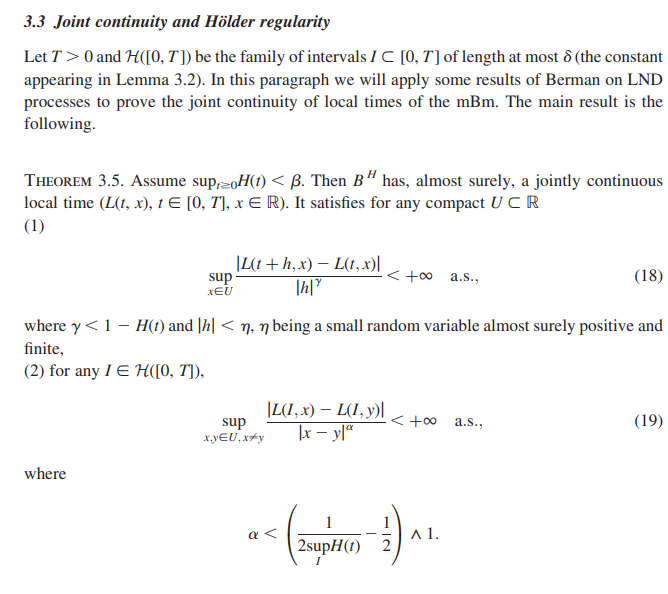and
from "Occupation time problems for fractional Brownian motion and some other self-similar processes"
Suppose by symmetry that fBM starts at $x<a$. So consider $A_{r}=(a,a+r)$ and $A_{-r}=(a-r,a)$ and their occupation times during $I=:[\sigma(a),\sigma(a)+\epsilon]$ for $\epsilon<\delta$ from the theorem
$$\mu_{I}(A_{r})=\int_{A_{r}}\ell_{I,x}dx, \mu_{I}(A_{-r})=\int_{A_{r}}\ell_{I,x}dx.$$
Now by contradiction suppose that only one of those occupation time is zero, say $ \mu_{I}(A_{-r})=0$. Both occupation measures cannot be both zero because that would imply that fBM either jumps (but it is continuous) or it is constant equal to $a$ but in fact it is nowhere differentiable; this can be proved with local time continuity as in here. So we get $ \mu_{I}(A_{r})>0$.
However, we use bound $\ell_{x,I}\geq \ell_{x+r,I}-c|I|^{1-H(1+\delta)}r^{\delta}$ to get
$$0= \mu_{I}(A_{-r})> \mu_{I}(A_{r}) -c|I|^{1-H(1+\delta)}r^{\delta+1}.$$
By taking large enough $r$ (eg. $r>c_{Holder}\epsilon^{H-s}$ for $s>0$), we get $ \mu_{I}(A_{r})=|I|=\epsilon>0$ and so
$$0= \mu_{I}(A_{-r})> \epsilon-c_{1}\epsilon^{1-H(1+\delta)}r^{\delta+1}.$$
Since $\epsilon>c\vee 1$ for some large random $c$ depending on $c_{Holder},c_{1}$, we get $\epsilon^{H}>r>c_{Holder}\epsilon^{H-s}$ and so
$$1-c_{1}(\frac{r}{\epsilon^{H}})^{1+\delta}=1-c_{1}(c_{Holder}\epsilon^{-s_{1}})^{1+\delta}>0$$
for $s_{1}<s$.