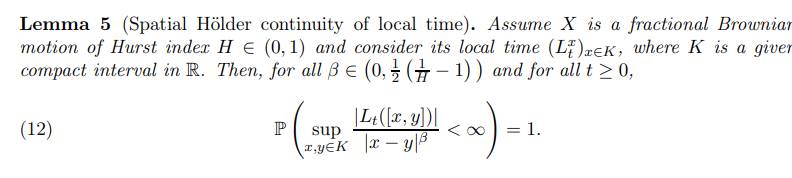The local time $\ell_{x,t}$ of fractional Brownian motio is continuous eg."A uniform result for the dimension of fractional Brownian motion level sets"
Suppose by symmetry that fBM starts at $x<a$. So consider $A_{r}=(a,a+r)$ and $A_{-r}=(a-r,a)$ and their occupation times
$$\mu_{t}(A_{r})=\int_{A_{r}}\ell_{x,t}dx, \mu_{t}(A_{-r})=\int_{A_{-r}}\ell_{y,t}dy.$$
We see that $\mu_{\sigma(a)+\epsilon}(A_{r})-\mu_{\sigma(a)}(A_{r})$ is the occupation time of the set $A_{r}$ between the times $[\sigma(a),\sigma(a)+\epsilon]$ because $\mu_{\sigma(a)}(A_{r})=0$. Similarly for $\mu_{\sigma(a)+\epsilon}(A_{-r})-\mu_{\sigma(a)}(A_{-r}).$
Now by contradiction suppose that only one of those occupation time is zero, say $\mu_{[\sigma(a),\sigma(a)+\epsilon]}(A_{-r})=0$ i.e. $\mu_{\sigma(a)+\epsilon}(A_{-r})=\mu_{\sigma(a)}(A_{-r})=\sigma(a)$. Both occupation measures cannot be both zero because that would imply that fBM either jumps (but it is continuous) or it is constant equal to $a$ but in fact it is nowhere differentiable; this can be proved with local time continuity as in here. So we get $\mu_{[\sigma(a),\sigma(a)+\epsilon]}(A_{-r})=\epsilon$.
However, by continuity we use bound $\ell_{x}\geq \ell_{x+r}-cr^{\beta}$
$$0=\int_{A_{-r}}\ell_{x,\sigma(a)+\epsilon}dx-\sigma(a)>\mu_{\sigma(a)+\epsilon}(A_{r})-\sigma(a) -cr^{\beta+1}=\epsilon-cr^{\beta+1}.$$ So by taking $r$ small enough we get a contradiction.