In spirit it is similar to the deterministic PDEs where one needs to use the regularity of the generator and various embedding theorems. At least in the linear case with additive noise
$$d X(t) = (AX(t) + f (t))dt + BdW(t),$$
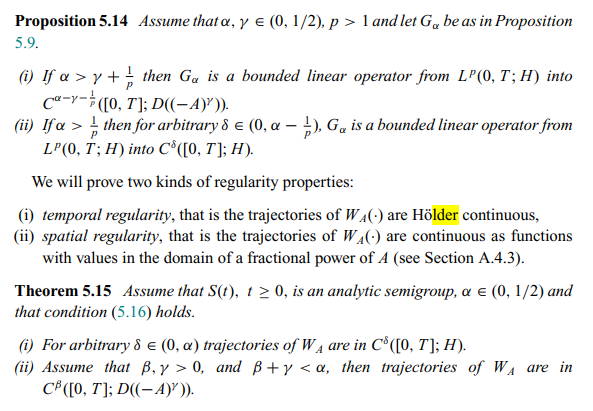
where $A: D(A) \subset H \to H$ and $B : U \to H$ are linear operators and $f$ is an $H-$valued stochastic process, in "Stochastic Equations in Infinite Dimensions" the authors cover a main regularity result. For generator $A$, let $S$ be the semigroup generated by it, then
$$X(t) = S(t)X_0 + \int_{0}^{t}S(t − s) f (s)ds + \int_{0}^{t} S(t − s)BdW(s),$$
solves the above linear spde. For $W_{A}(t):= \int_{0}^{t} S(t − s)BdW(s)$, they show in theorems 5.14,5.15 a Holder regularity for it