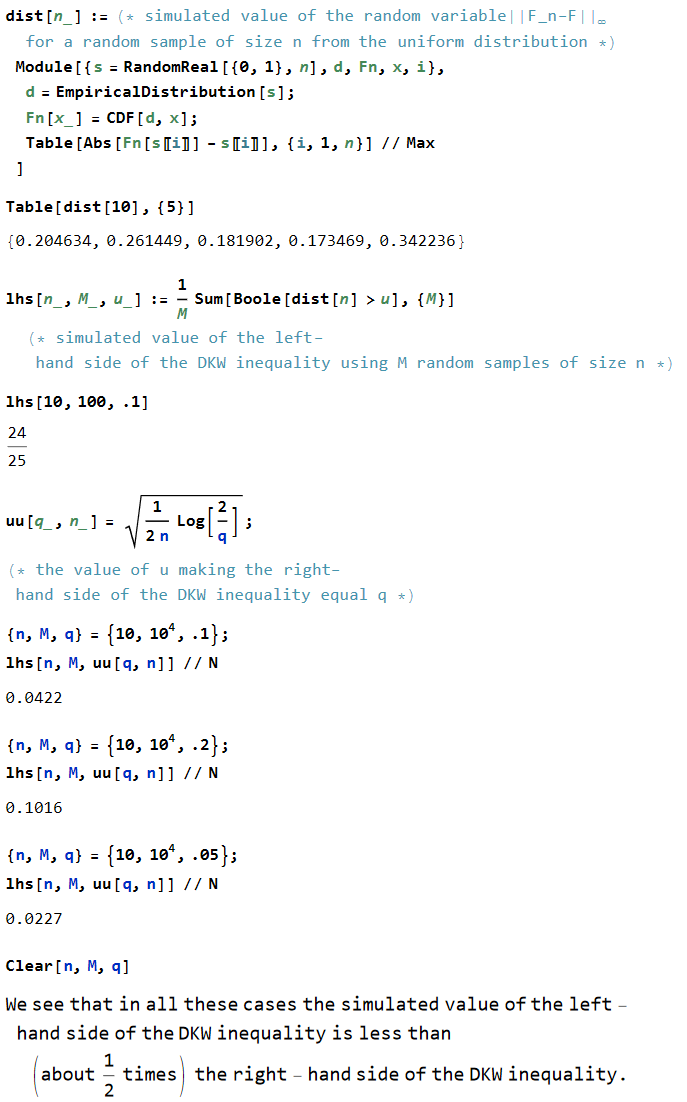
The DKW inequality is $$P(\|F_n-F\|_\infty>u)\le2e^{-2nu^2}$$ for $u>0$, where $F$ is the true cdf and $F_n$ is the empirical cdf.
Your inequality is different. It is something like this: $$P(\|F_n^{-1}-F^{-1}\|_\infty>u)\le2e^{-2nu^2}$$ for $u>0$. (It is unclear to me how your (sample?) quantiles $q_i$ are defined; I cannot read your code.)
Below is an image of a Mathematica notebook confirming the DKW inequality: