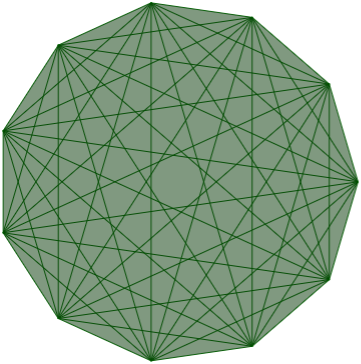
Consider a regular $n$-gon of side length $1$ with diagonals. Here is an example with $n=11$ (from geogebra applet).

I've been trying to find, in terms of $n$, bounds on the area of the largest cell, excluding the centre cell when $n$ is odd.

It seems that, for every value of $n$, the largest non-centre cell has roughly similar area as the outermost cell, whose area is $\frac{1}{4}\tan{\frac{\pi}{n}}\approx\frac{\pi}{4n}$.

That is, $(\text{area of largest non-centre cell})$ is on the order of $n^{-1}$.

(This claim has numerical evidence: the first graph in this answer shows that, in a regular $n$-gon of radius $1$ with diagonals, up to $n=200$, the radius of the largest disk that fits within a cell, is approximately $3n^{-1.5}$; scaling to a regular $n$-gon of side length $1$, the largest disk would have an area of approximately $\frac{9}{4\pi n}$.)

Question:

In a regular $n$-gon of side length $1$ with diagonals, what is the infimum and supremum of $n\times(\text{area of largest non-centre cell})$ ?

(The number of cells is approximately $\frac{1}{24}n^4$ for large $n$.)

Cross-posted on MSE.