The LHS might not make sense if g is very irregular.
Consider, pth-Holder function $g(x)=x^{1/p}$ for $p<1$ and $\frac{1}{p}$ odd integer and Brownian motion $X_{t}=B_{t}$, then the process $g(X_{t})= (B_{t})^{1/p}$ for $p<\frac{1}{3}$ is not even in the usual regime for rough integrals (Uniqueness of solutions of Young differential equations). So we would also need to formulate the LHS in some way.
Generally, we also need $C^2$/Convex in order to apply change of variables see here https://almostsuremath.com/2020/10/12/the-ito-tanaka-meyer-formula/
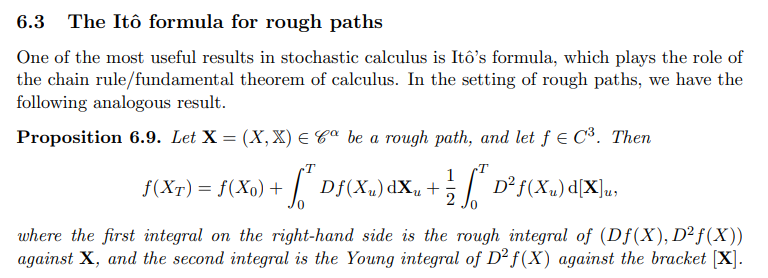
In even weaker settings of rough paths, there is still an Ito formula (In Proposition 6.9 "Rough Path Theory" by Andrew L. Allan).