You might also want to see: Alspach, B., Hamilton cycles in cubic Cayley graphs on dihedral groups, Ars Comb. 28, 101–108 (1989). Zbl 0722.05047. PDF available at ResearchGate.
Let $C_n$ and $P_n$ denote the cycle and path of length $n$, respectively.
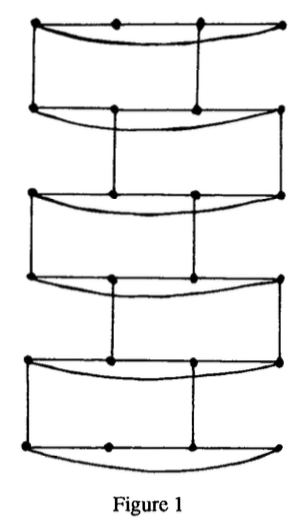
2.1 Definition: The brick product of $C_n$ with $P_m$, $m \geq 1$, is denoted $C_n^{[m+1]}$ and is defined as follows. Let $V(C_n) = \{ u_1, u_2, \dots, u_n \}$, $E(C_n) = \{ u_1 u_2, u_2 u_3, \dots, u_n u_1 \}$, $V(P_m) = \{ v_1, v_2, \dots, v_{m+1} \}$, and $E(P_m) = \{ v_1 v_2, \dots, v_m v_{m+1} \}$. The vertex-set of $C_m^{[m+1]}$ [typo: should be $C_n^{[m+1]}$] is the cartesian product $V(C_n) \times V(P_m)$. The edge-set consists of all pairs of the form $(u_i, v_t) (u_i, v_{t+1})$, where $i+t \equiv 0 \pmod{2}$, $i = 1, 2, \dots, n$, and $t = 1, 2, \dots, m$. The brick product $C_4^{[5]}$ is shown in Figure 1.