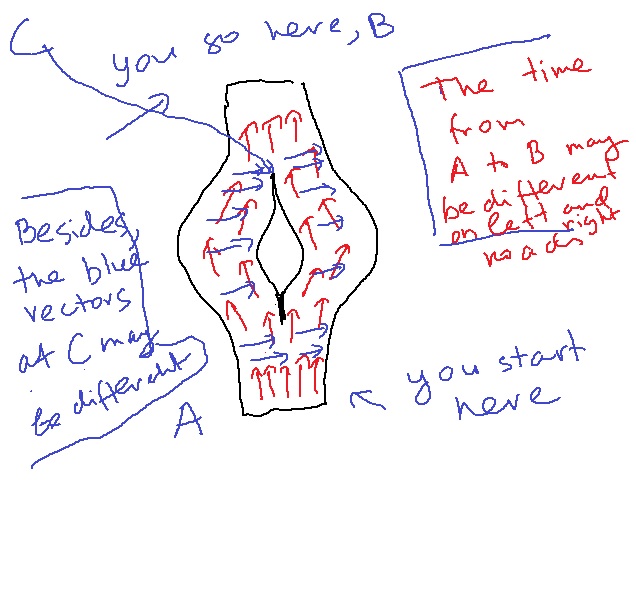
The picture above gives a counterexample to your hope. It is in dimension 2 but there is no problem to make it in any higher dimension. The commuting vector fields are red and blue, the compact is not simply connected and the ``hole'' is such that at the point C the boundary curves coincide in jets.
The caption on the right (in red) says that if you go along the red vector field along the hole you may come to the other side in different times (say, left was is faster thab the right way). If you have a commutative blue vector field which can be extended to a hole than it is not possible, since the blue vector field connects the left and the right ways.
The caption on the left (blue) says that the pushforward of the blue vector field from the bottom to the top along the orbits of the red vector field must not match, in general. Indeed, on the top the pushforward of the blue vector field along the flow of the red vector field in the right side may be twice the pushforward of blue vector field along the flow of the read vector field in the left side, which is also not possible if the flow is extended. Note that if vector field $v$ and $u$ commute, then for any constant $C$ the vector fields $v$ and $Cu$ also commute