Here's a few empirical results that might be helpful, particularly in lending credence towards some of the theories suggested in other posts. I ran 10,000 simulations, and for each one, broke the stick 10,000 times, tracking the longest portion at each step. Here's the results:
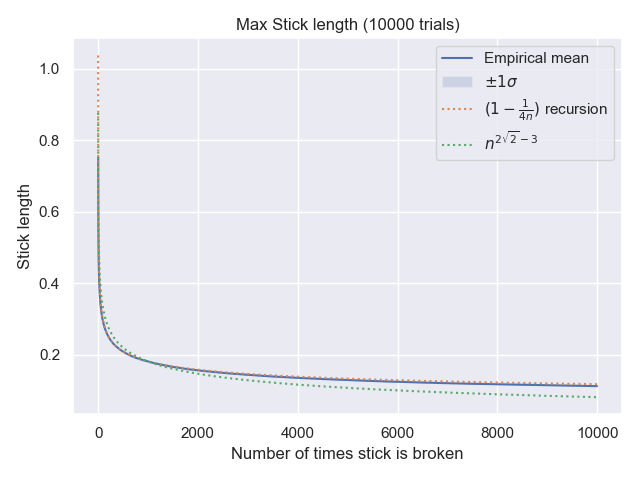
and the same data plotted on a log scale:
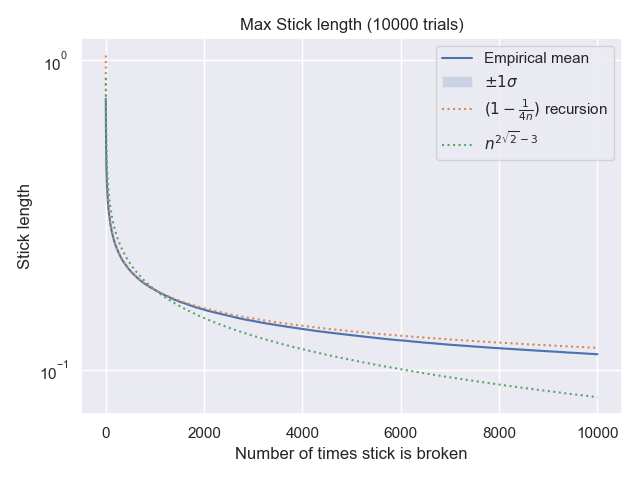
You may notice a highlighted $\pm 1 \sigma$ region mentioned on the legend; the corresponding confidence interval is so tight that it's hard to see.
I added two dotted lines corresponding to two rates of decay mentioned in other posts: $d(n) \propto n^{2\sqrt{2}-3}$ and $d(n) \propto (1-\frac{1}{4n-4})d(n-1)$.
The two hypotheses are intended as asymptotic descriptions, so if we plot them directly, they have large and distracting offsets. I "fixed" that by adding or subtracting a constant to make the plots intersect the empirical mean at $x=1000$.
The figures would seem to suggest that the $1-\frac{1}{4n}$ recursion may be a tighter fit.
Incidentally, we can compute our own version of the expected length after the 500th split. I ran 1 million simulations and observe an empirical mean of $0.2076537 \pm 0.0001231$. (The $\pm$ part is one standard deviation for the estimate.)
