This sequence, say $(a_1,a_2,\dots)$, is the increasing enumeration of the set (say $S$) of natural numbers $k$ such that each of the numbers $k-1,k,k+1$ is semiprime. So, the problem seems to be of the same flavor as the twin prime conjecture.
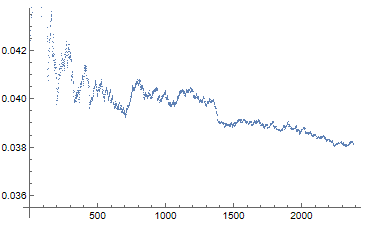
Here is the graph $\{(n,\frac{n\,\ln n\,\ln\ln n}{a_n})\colon n=2,3,\dots,\ a_n\le10^6\}$:
This graph suggests that $a_n\asymp n\,\ln n\,\ln\ln n$ and hence $\sum_n\frac1{a_n}=\infty$. At least, the graph suggests that $a_n\ge c n\,\ln n\,\ln\ln n$ for some real $c>0$ and all natural $n$, and then the set $S$, of all semiprimes $k$ "sandwiched" between semiprimes $k-1,k+1$, would be infinite.
So, it seems unclear if this problem is easier than the twin prime conjecture.