$\DeclareMathOperator{\conv}{conv} \DeclareMathOperator{\kk}{\mathbb{K}} \DeclareMathOperator{ld}{Ld} \DeclareMathOperator{\mv}{MV} \DeclareMathOperator{\supp}{supp}$Since there is no answer yet, and partly in answer to a comment to the OP by Loren, let me add as answer my peeve with the usual formulations of Bernstein–Kushnirenko theorem. The point is the following: starting with fixed finite subsets $A_1, \dotsc, A_n$ of $\mathbb{Z}^n$, the original formulation is a statement about the number of solutions of polynomials $f_1, \dotsc, f_n$ such that $\supp(f_i) \subseteq A_i$, and it gives a precise characterization of when this number achieves the maximum. However, in almost every place you look, the statement presented is about polynomials $f_i$ such that $\supp(f_i) = A_i$.
Of course $\supp(f_i) = A_i$ is the case people are concerned about in most cases. But the original formulation is clearly stronger (and as far as I remember, has practically the same proof as required for the weaker statement). In addition, the non-degeneracy condition in the stronger (and, let me stress again, original) formulation almost immediately gives a precise characterization for when $\mv(P_1, \dotsc, P_n) < \mv(Q_1, \dotsc, Q_n)$ for $P_i \subseteq Q_i$. The first observation of this seems to be due to Maurice Rojas (A convex geometric approach to counting the roots of a polynomial system) in 1994, but it was rediscovered very recently in 2017 (Bihan, Soprunov, Criteria for strict monotonicity of the mixed volume of convex polytopes). The fact that it needed to be "rediscovered" is I think at least partly due to the unawareness of the complete statement of Bernstein–Kushnirenko theorem among its users. In fact I only learned about the complete statement after I started writing the second draft of a book on Bernstein–Kushnirenko theorem and wanted to characterize the strict monotonicy of mixed volume as an application. (To be pedantic — Bernstein–Kushnirenko theorem directly gives the strict monotonicity only for rational polytopes. But the statement about all polytopes then immediately follows from the continuity of mixed volumes with respect to the Hausdorff Measure.)
Also, with respect to the original formulation of non-degeneracy conditions, the set of non-degenerate systems is Zariski open. In the usual formulation, if you are not very careful or unless you allow for some not-very-natural modifications, the set of non-degenerate systems turns out to contain a (nonempty) Zariski open subset, but might not be open itself.
Enough rant; here is the correct statement (see the book mentioned above for a proof): fix an algebraically closed field $\kk$.
Given finite subsets $A_i$ of $\mathbb{Z}^n$ and Laurent polynomials $f_i$ with $\supp(f_i) \subseteq A_i$, $i = 1, \dotsc, n$, the number (counted with multiplicity) of isolated solutions of $f_1, \dotsc,$ $f_n$ on $(\kk\setminus\{0\})^n$ is at most $\mv(\conv(A_1), \dotsc,\conv(A_n))$. This maximum is attained if and only if the following holds:
For every nonzero weighted degree $\omega$ on $\kk[x_1, \dotsc, x_n]$, there is no common zero on $(\kk \setminus \{0\})^n$ of the "$A_i$-supported leading forms" of $f_i$.
The "$A$-supported leading form" $\ld_{A, \omega}(f)$ of a Laurent polynomial $f$ supported in a finite (or compact) subset $A$ of $\mathbb{R}^n$ is defined as follows: first write $\ld_\omega(A)$ for the "leading $\omega$-face" of $A$, i.e. the set of all $\alpha \in A$ such that $\langle \omega, \alpha \rangle = \max\{\langle \omega, \alpha'\rangle: \alpha' \in A\}$. If $f = \sum_\alpha c_\alpha x^\alpha$, then $$\ld_{A, \omega}(f) := \sum_{\alpha \in \ld_\omega(A)} c_\alpha x^\alpha$$ In particular, if $\supp(f) \cap \ld_\omega(A) = \emptyset$ then $\ld_{A, \omega}(f) = 0$.
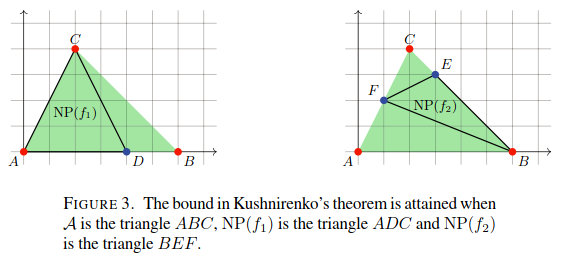
Consider e.g. $f_1 = 1 + x^4 + x^2y^4$, $f_2 = xy^2 + x^3y^3 + x^6$, and $A$ is the set of integral points in the triangle $ABC$ in the picture above (reproduced from the book mentioned above). Even though the Newton polygons of both $f_i$ are proper subsets of $A$, it is immediate to check that the Bernstein–Kushnirenko non-degeneracy condition is satisfied with $A_1 = A_2 = A$, and therefore the number of solutions of $f_1 = f_2 = 0$ in $(\kk\setminus{0})^2$ is $\mv(\conv(A), \conv(A)) = 2\operatorname{Area}(\conv(A)) = 24$.