The distribution of the spacings of the eigenvalues of the GUE matrix $B$ is given by the Wigner surmise, $$p(s)=(32s^2/\pi^2) \exp \left(-4 s^2/\pi\right),$$ where the spacing $s>0$ of adjacent eigenvalues is measured is units of the mean spacing. The spacing distribution of the sum $A+B$, with $A$ a fixed symmetric matrix, is assumed to be well described by $p(s)$. There is some evidence for that, see Universality of the local spacing distribution in certain ensembles of hermitian Wigner matrices.
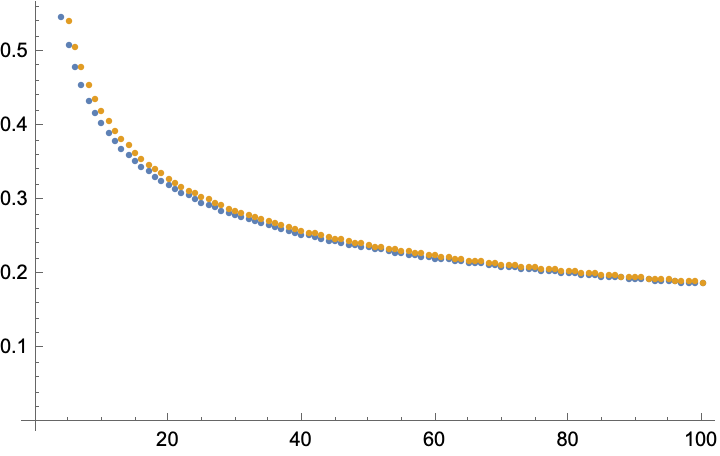
Eigenvalues that are not adjacent are only weakly correlated, I will assume that different spacings are independent. Then the expectation value of the minimal spacing $s_{\rm min}$ for $n\times n$ matrices is given by $$\mathbb{E}[s_{\rm min}]=\int_0^\infty [1-c(s)]^n\,ds,$$ with $c(s)=\int_0^s p(s')ds'$ the cumulative spacing distribution. This integral does not have a closed form expression, for large $n$ it tends to $$\mathbb{E}[s_{\rm min}]\rightarrow \Gamma(4/3)n^{-1/3},\;\;n\gg 1.$$ Below is a plot up to $n=100$, gold is the integral, blue is the asymptotic.
It would be of interest to see if the $n^{-1/3}$ scaling survives an exact calculation (not making the assumption of independent spacings).