Inspired by a discussion with Neil Strickland I am very interested to hear of explicit examples (one per answer, please), as follows.
A compact Riemann surface can be presented in many different ways. For example:
- A smoothly embedded embedded surface in the three-sphere $S^3$.
- A smooth projective curve (say cut out of $\mathbb{CP}^2$ by a single equation).
- A quotient of the hyperbolic plane by some fuchsian group.
For each of these "ways" we can accept some minor modifications.
We prefer embeddings into $S^3$ (or the three-torus $\mathbb{T}^3$) because we want to actually "see" the surface. However giving the surface as a level-set of a nice function, or via some other nice analytical construction (for example as a minimal surface with symmetries), is also welcome.
I am not algebraic enough to deform the condition given in (2) - I hope some reader will suggest the correct modifications.
We also accept quotients of $\mathbb{C}$ by a lattice $\mathbb{Z} + \mathbb{Z}\omega$. We also accept tilings of the upper half-plane as long as the tiling has no "moduli" (or has enough explicit side conditions) so that the fuchsian group can be deduced, with sufficient amount of hyperbolic trig). We may also modify (3) in another way - for example giving square-tiled surfaces or more generally surfaces given by gluing explicitly described polygons in $S^2$, $\mathbb{E}^2$, or $\mathbb{H}^2$.
Question: Give explicit examples of pairs (or preferably triples) of isomorphic Riemann surfaces of the above types.
We first dispose of the trivial example of the sphere. Here (1) and (3') are addressed by saying "the round sphere". (2) is addressed by saying (for example) "$x + y + z = 0$".
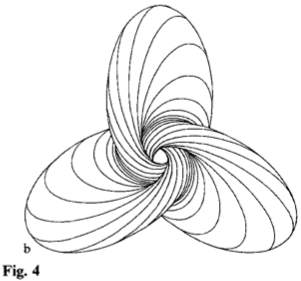
As an actual example, we have tori. For (3), we specify $\omega$ and thus the lattice $\mathbb{Z} + \mathbb{Z}\omega$. For (2), we have the Eisenstein series giving the modular invariants. For (1) we have the Hopf tori embedded in $S^3$ (and thus, after stereographic projection, embedded in three-space). Pinkall [Inventiones, 1985] defines these, and has explicit constructions (see Figure 4b (reproduced below) and Figure 6b). See also Figure 5 of Sullivan [Bridges, 2011].
I give further examples in the comments here but please see, in addition, Strickland's talk.