https://userpages.monmouth.com/~colonel/nrectcover/index.html

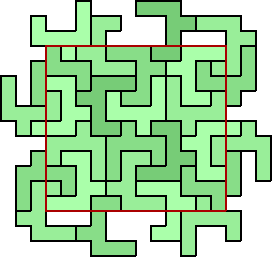
For a polyomino with no holes that cannot tile the plane, we may ask what are the maximal rectangles and infinite strips that it can cover without overlapping, allowing the tiles to extend beyond the region's perimeter.

example from this site:

But can there be such a polyomino that can cover an arbitrarily large square, but not the whole plane? And is this even possible for an arbitrary tile at all? The answer is probably no, but why exactly.

In other words, can polyomino which cannot tile the plane produce infinite sequence of squares with increasing edge length?